Single TF

$$\newcommand{\kon}{k_{\mathrm{on}}} \newcommand{\koff}{k_{\mathrm{off}}}$$

There exist two mathematical formalisms to describe the probabilities of molecular configurations at equilibrium: thermodynamics and kinetics. In the kinetics formalism, the system transits between configurational states according to rate parameters contained in differential equations, which can describe not just the system's equilibrium but also its trajectory towards it, or the kinetics of non-equilibrium systems. In the thermodynamics/statistical mechanics formalism, we posit that a system will occupy configurations with lower energy, and use the Boltzmann distribution to estimate the proportion of time the system spends in each state, at equilibrium. The thermodynamics formalism is limited to describing equilibrium state probabilities, but it does so with fewer parameters.

We'll derive an expression for the probability of a single TFBS' occupancy with both formalisms, but proceed with the thermodynamic description for more elaborate configurations. It will become clear why that is preferable.

Kinetics

Most derivations of the probability of single TFBS occupancy at equilibrium employ a kinetics formalism, so we'll walk through that first, and then explore the analog in the thermodynamics description. In the kinetics description, the parameters are rates.

$$ \mathrm{TF} + \mathrm{TFBS} \underset{\koff}{\overset{\kon}{\rightleftarrows}} \mathrm{TF\colon TFBS} $$

The natural rates are the rate of TF binding $\kon$ and unbinding $\koff$. Equilibrium is reached when binding and unbinding are balanced:

$$\frac{d[\mathrm{TF\colon TFBS}]}{dt} = k_{\mathrm{on}}[\mathrm{TF}][\mathrm{TFBS}] - k_{\mathrm{off}}[\mathrm{TF\colon TFBS}] = 0 \text{ at equilibrium}$$ $$k_{\mathrm{on}}[\mathrm{TF}]_{\mathrm{eq}}[\mathrm{TFBS}]_{\mathrm{eq}} = k_{\mathrm{off}}[\mathrm{TF\colon TFBS}]_{\mathrm{eq}}$$ $$\text{(dropping eq subscript) }[\mathrm{TF\colon TFBS}] = \frac{k_{\mathrm{on}}[\mathrm{TF}][\mathrm{TFBS}]}{k_{\mathrm{off}}} = \frac{[\mathrm{TF}][\mathrm{TFBS}]}{k_{d}}$$

where $k_{d} = \frac{\koff}{\kon}$ is called the dissociation constant (or equilibrium constant). We'd like to determine the probability of finding the TFBS occupied, i.e. the fraction of time it spends in the bound state. That fraction is $\frac{[\mathrm{bound}]}{([\mathrm{unbound}] + [\mathrm{bound}])} = \frac{[\mathrm{TF\colon TFBS}]}{([\mathrm{TFBS}] + [\mathrm{TF\colon TFBS}])}$. Define the denominator as $[\mathrm{TFBS}]_{0} = [\mathrm{TFBS}] + [\mathrm{TF\colon TFBS}]$ so that $[\mathrm{TFBS}] = [\mathrm{TFBS}]_{0} - [\mathrm{TF\colon TFBS}]$ and substitute:

$$[\mathrm{TF\colon TFBS}] = \frac{[\mathrm{TF}]([\mathrm{TFBS}]_{0} - [\mathrm{TF\colon TFBS}])}{k_{d}}$$ $$[\mathrm{TF\colon TFBS}](k_d + [\mathrm{TF}]) = [\mathrm{TF}][\mathrm{TFBS}]_{0}$$ $$\frac{[\mathrm{TF\colon TFBS}]}{[\mathrm{TFBS}]_{0}} = \frac{[\mathrm{TF}]}{k_d + [\mathrm{TF}]}$$

Note: We could also ask for this expression in terms of $[\mathrm{TF}]_0 = [\mathrm{TF}] + [\mathrm{TF\colon TFBS}]$ however, since we’re considering a single TFBS, $[\mathrm{TF\colon TFBS}]$ is at most 1, and so $[\mathrm{TF}]_0 \approx [\mathrm{TF}]$. In instances of ligand-receptor binding in which that approximation cannot be made, the fraction bound is a messy quadratic. Derivation here.

Thermodynamics

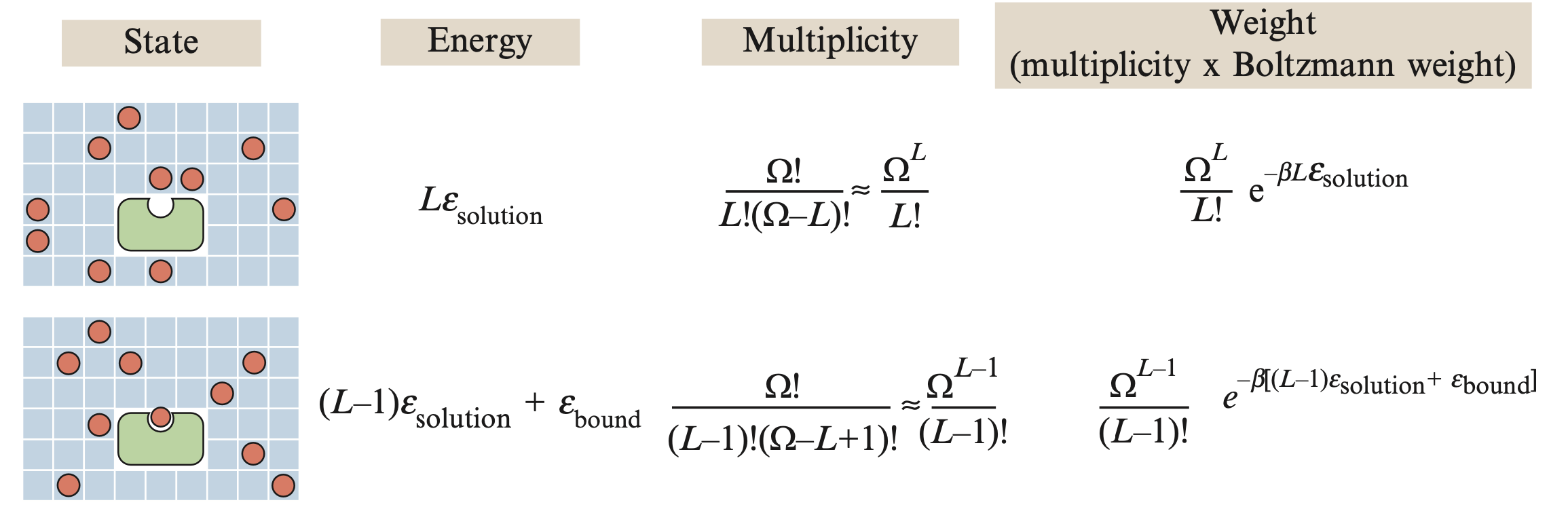
In the thermodynamics description, the parameters are Gibbs free energies $\Delta G$. Let's follow the derivation from Physical Biology of the Cell (pp. 242) and consider the number microstates underlying each of the of bound and unbound macrostates, and their energies.

In order to count microstates, we imagine distributing $L$ TF molecules across a space-filling lattice with $\Omega$ sites. The energy of a TF in solution is $\varepsilon_{\mathrm{solution}}$ and the energy of a bound TF is $\varepsilon_{\mathrm{bound}}$. $\beta$ is the constant $1/k_b T$ where $k_b$ is Boltzmann's constant and $T$ is the temperature.

StateEnergyMultiplicityWeight
$A \cdot A_s$ $\frac{\Omega!}{(\Omega - A)!A!} \approx \frac{\Omega^{A}}{A!}$ $\frac{\Omega^{A}}{A!} \cdot e^{-\beta \left[ A \cdot A_s \right]}$
$(A - 1) A_s + A_b$ $\frac{\Omega!}{(\Omega - (A - 1))!(A-1)!B!} \approx \frac{\Omega^{A-1}}{(A-1)!}$ $\frac{\Omega^{A-1}}{(A-1)!} \cdot e^{-\beta \left[ (A - 1) A_s + A_b \right]}$

In our case, the number of microstates in the unbound macrostate is $\frac{\Omega !}{L!(\Omega -L)!}\approx \frac{\Omega^L}{L!}$ and they each have energy $L \cdot \varepsilon_s$. The number of microstates in the bound macrostate is $\frac{\Omega !}{(L-1)!(\Omega -(L+1))}\approx \frac{\Omega^{(L-1)}}{(L-1)!}$ and they each have energy $(L-1) \varepsilon_s + \varepsilon_b$.

The Boltzmann distribution describes the probability of a microstate as a function of its energy: $p(E_i) = e^{-\beta E_i}/Z$ where $Z$ is the "partition function" or simply $\sum_i e^{-\beta E_i}$ the sum of the weights of the microstates, which normalizes the distribution. In our case:

$$Z(L,\Omega)=\left(\colorbox{LightCyan}{$ \frac{\Omega^L}{L!} e^{-\beta L \varepsilon_s}$}\right) + \left(\colorbox{Seashell}{$\frac{\Omega^{(L-1)}}{(L-1)!} e^{-\beta [(L-1) \varepsilon_s + \varepsilon_b]}$}\right)$$

With that expression in hand, we can express the probability of the bound macrostate, $p_b$:

$$p_b=\frac{ \colorbox{Seashell}{$\frac{\Omega^{(L-1)}}{(L-1)!} e^{-\beta [(L-1) \varepsilon_s + \varepsilon_b]}$}}{\colorbox{LightCyan}{$\frac{\Omega^L}{L!} e^{-\beta L \varepsilon_s}$} + \colorbox{Seashell}{$\frac{\Omega^{(L-1)}}{(L-1)!} e^{-\beta [(L-1) \varepsilon_s + \varepsilon_b]}$}} \cdot \color{DarkRed}\frac{\frac{\Omega^L}{L!}e^{\beta L \varepsilon_s}}{\frac{\Omega^L}{L!}e^{\beta L \varepsilon_s}} \color{black} = \frac{(L/\Omega)e^{- \beta \Delta \varepsilon}}{1+(L/\Omega)e^{- \beta \Delta \varepsilon}} $$

Where we have defined $\Delta \varepsilon = \varepsilon_b - \varepsilon_s$. $L/\Omega$ is really just a dimensionless TF concentration, which we'll hand-wave as being equivalent to $[\mathrm{TF}]$, which leaves us with an expression suspiciously similar to the one we derived from the kinetics formalism:

$$p_b = \frac{[\mathrm{TF}]e^{-\beta \Delta \varepsilon}}{1+[\mathrm{TF}]e^{-\beta \Delta \varepsilon}} \cdot \color{DarkRed}\frac{e^{\beta \Delta \varepsilon}}{e^{\beta \Delta \varepsilon}} \color{black} = \frac{[\mathrm{TF}]}{e^{\beta \Delta \varepsilon}+[\mathrm{TF}]}$$

From which we recapitulate an important correspondence between kinetics and thermodynamics at equilibrium: $ k_d = e^{\beta \Delta \varepsilon} = e^{\Delta \varepsilon / k_bT} $ more commonly written for different units as $k = e^{-\Delta G / RT}$.

The takeaway is that both the kinetics and thermodynamics formalisms produce an equivalent expression for the probabilities of each of the bound and unbound configurations, parameterized respectively by $k_d$ and $\Delta G$.

References:

Sample Values

In order to compute probabilities like $p_b$, we need concrete TF concentrations $[\mathrm{TF}]$ and binding affinities (either $k_d$ or $\Delta G$). What are typical intranuclear TF concentrations and binding affinities?

Concentrations

A typical human cell line, K562s, have a cellular diameter of 17 microns. (BioNumbers)

def sphere_volume(d):
    return 4/3*np.pi*(d/2)**3

K562_diameter_microns = 17
K562_volume_micron_cubed = sphere_volume(K562_diameter_microns)
print(f'K562 volume: {round(K562_volume_micron_cubed)} μm^3')

K562 volume: 2572 μm^3

A typical expressed TF has a per-cell copy number range from $10^3$ - $10^6$. (BioNumbers)

copy_number_range = [1e3, 1e6]
N_A = 6.02214076e23

def copy_number_and_cubic_micron_volume_to_molar_concentration(copy_number, volume=K562_volume_micron_cubed):
    return (copy_number / N_A) / (volume * (1e3 / 1e18))  # 1000 Liters / m^3; 1e18 μm^3 / m^3

lower_end_molar = copy_number_and_cubic_micron_volume_to_molar_concentration(copy_number_range[0], K562_volume_micron_cubed)
upper_end_molar = copy_number_and_cubic_micron_volume_to_molar_concentration(copy_number_range[1], K562_volume_micron_cubed)

lower_end_nanomolar = lower_end_molar / 1e-9
upper_end_nanomolar = upper_end_molar / 1e-9

print('If TF copy numbers range from 1,000-1,000,000, then TF concentrations range from', str(round(lower_end_nanomolar))+'nM', 'to', str(round(upper_end_nanomolar))+'nM')

If TF copy numbers range from 1,000-1,000,000, then TF concentrations range from 1nM to 646nM

We might also like a distribution over this range. Let's posit a lognormal, where $10^3$ and $10^6$ are the 3σ from the mean, which is $10^{4.5}$. Then $\sigma = 10^{0.5}$

Affinities

What are typical TF ΔG's of binding? How about the $\koff$ rates and half lives?

  • We can use the prior knowledge that dissociation constants should be in the nanomolar regime (BioNumbers).
  • We can use the relation that $\Delta G = -k_b T \cdot \ln(k_d)$ (Plugging in 310°K (human body temp) and the Boltzmann constant $k_b$ in kcal/Mol)
  • We use the approximation that $\kon$ is ~$10^5 / $ Molar $ \times $ sec (Wittrup)
T = 310
k_b = 3.297623483e-24 * 1e-3  ## cal/K * kcal/cal
kbT = k_b*T*N_A
kbT  ## in 1/Mol -- an unusual format

k_on = 1e5

def nanomolar_kd_from_kcal_ΔG(ΔG): return exp(-ΔG/kbT) * 1e9
def kcal_ΔG_from_nanomolar_kd(K_d): return -kbT*ln(K_d*1e-9)
def k_off_from_nanomolar_kd(k_d): return (k_d*1e-9) * k_on
def half_life_from_kd(k_d): return ln(2) / ((k_d*1e-9) * k_on)
$\Delta G$ $\kon$ $\koff$ $t_{1/2}$
$k_d$
1 12.757685 1e5 / (M * s) 0.0001 0 days 01:55:31.471805599
10 11.340164 1e5 / (M * s) 0.0010 0 days 00:11:33.147180560
100 9.922644 1e5 / (M * s) 0.0100 0 days 00:01:09.314718056
1000 8.505123 1e5 / (M * s) 0.1000 0 days 00:00:06.931471806

We learn that an order of magnitude residence time difference results from just 1.4 extra kcal/Mol, and that TF half lives range from about 5s to about 2h. Let's once again posit a distribution of affinities to sample from (defined on $k_d$):

With those concrete TF concentrations and dissociation constants, we can finally plot our function $p_b = \frac{[\mathrm{TF}]}{e^{\beta \Delta \varepsilon}+[\mathrm{TF}]}$.

@np.vectorize
def fraction_bound(TF, ΔG):
    '''TF in nanomolar'''
    return TF / (TF + nanomolar_kd_from_kcal_ΔG(ΔG))

(Note that both $[\mathrm{TF}]$ and $k_d$ are plotted in log space, but $p_b$ is linear.)

Multiple TFs: Direct Cooperativity & Competition

Suppose now that two TFs bind adjacent segments of DNA in such a way that the binding of either facilitates (or hinders) the binding of the other. We call this direct cooperativity (and competition). We'll focus first on cooperativity.

Cooperativity

As before, the statistical mechanics formalism entails enumerating the configurations, their multiplicities, and their energies. We'll call the TFs A and B. We'll denote their counts as $A$ and $B$. The energy of a TF in solution will once more be $A_s$ and bound to its cognate TFBS $A_b$. The energy of cooperativity will be $C_{AB}$.

StateEnergyMultiplicityWeight
$A \cdot A_s + B \cdot B_s$ $\frac{\Omega!}{(\Omega - A - B)!A!B!} \approx \frac{\Omega^{A+B}}{A!B!}$ $\frac{\Omega^{A+B}}{A!B!} \cdot e^{-\beta \left[ A \cdot A_s + B \cdot B_s \right]}$
$(A - 1) A_s + A_b + B \cdot B_s$ $\frac{\Omega!}{(\Omega - (A - 1) - B)!(A-1)!B!} \approx \frac{\Omega^{A+B-1}}{(A-1)!B!}$ $\frac{\Omega^{A+B-1}}{(A-1)!B!} \cdot e^{-\beta \left[ (A - 1) A_s + A_b + B \cdot B_s \right]}$
$A \cdot A_s + (B - 1) B_s + B_b$ $\frac{\Omega!}{(\Omega - A - (B - 1))!A!(B-1)!} \approx \frac{\Omega^{A+B-1}}{A!(B-1)!}$ $\frac{\Omega^{A+B-1}}{A!(B-1)!} \cdot e^{-\beta \left[ A \cdot A_s + (B - 1) B_s + B_b \right]}$
$(A - 1) A_s + A_b + (B - 1) B_s + B_b + C_{AB}$ $\frac{\Omega!}{(\Omega - (A - 1) - (B-1))!(A-1)!(B-1)!} \approx \frac{\Omega^{A+B-2}}{(A-1)!(B-1)!}$ $\frac{\Omega^{A+B-2}}{(A-1)!(B-1)!} \cdot e^{-\beta \left[ (A - 1) A_s + A_b + (B - 1) B_s + B_b + C_{AB} \right]}$

The partition function is the sum of the weights:

$$ Z = \frac{\Omega^{A+B}}{A!B!} \cdot e^{-\beta \left[ A \cdot A_s + B \cdot B_s \right]} + \frac{\Omega^{A+B-1}}{(A-1)!B!} \cdot e^{-\beta \left[ (A - 1) A_s + A_b + B \cdot B_s \right]} + \frac{\Omega^{A+B-1}}{A!(B-1)!} \cdot e^{-\beta \left[ A \cdot A_s + (B - 1) B_s + B_b \right]} + \frac{\Omega^{A+B-2}}{(A-1)!(B-1)!} \cdot e^{-\beta \left[ (A - 1) A_s + A_b + (B - 1) B_s + B_b + C_{AB} \right]}$$

Which we can greatly simplify by multiplying the entire expression by the reciprocal of the "base state" weight, $\color{DarkRed}\frac{A!B!}{\Omega^{A+B}} \cdot e^{\beta \left[ A \cdot A_s + B \cdot B_s \right]}$, normalizing that weight to 1:

$$ Z = 1 + \frac{A}{\Omega} \cdot e^{-\beta \left[ A_b-A_s \right]} + \frac{B}{\Omega} \cdot e^{-\beta \left[ B_b-B_s \right]} + \frac{A \cdot B}{\Omega^2} \cdot e^{-\beta \left[ A_b-A_s+B_b-B_s+C_{AB} \right]}$$

Taking the definition $[A] = A/\Omega$ and $\Delta G_A = A_b-A_s$ produces:

$$ Z = 1 + [A] e^{-\beta \left[ \Delta G_A \right]} + [B] e^{-\beta \left[ \Delta G_B \right]} + [A][B] e^{-\beta \left[ \Delta G_A+\Delta G_B+C_{AB} \right]}$$

Then the probability of any state is just the weight of that state (scaled by the weight of the base state) divided by the partition function expression $Z$.

From the above, we notice the form of the expression for the weight of a configuration of N TFBSs:

$$ p_{\mathrm{config}} = \prod_{i \in \, \mathrm{bound \,TBFS}} \left( [\mathrm{TF}_{\mathrm{cognate}(i)}] \cdot e^{-\beta \left[ \Delta G_i + \sum_j c_{ij} \right]} \right) / Z$$

For numerical stability, we take the log of the unnormalized probability (that is, the weight) of configurations:

$$ \log(\tilde{p}_{\mathrm{config}}) = \sum_{i \in \, \mathrm{bound \,TBFS}} \left( \log([\mathrm{TF}_{\mathrm{cognate}(i)}]) - \beta \left[ \Delta G_i + \sum_j c_{ij} \right] \right) $$

Competition

Incorporating competition into our thermodynamic model is slightly more subtle than cooperativity, because we can imagine two types of competition

  • Two TFs which may both bind DNA at adjacent sites, causing a free energy penalty due to some unfavorable interaction.
  • Two TFs with overlapping DNA binding sites, which cannot physically be bound at the same time.

In the former case, the expression for $p_\mathrm{config}$ we had written before suffices, with values of $C_{AB}$ having both signs to represent cooperativity and competition. Nominally, the latter case also fits this formalism if we allow $C_{AB}$ to reach $-\infty$, but that would cause us headaches in the implementation. Instead, the weights of all those configurations which are not physical attainable, due to "strict" competitive binding between TFs vying for overlapping binding sites, are merely omitted from the denominator $Z$.

References:

Sample cooperativity values

In order to compute concrete probabilities of configurations, accounting for cooperativity and competition, we will need concrete energies. We'll take $C_{AB}$ to be distributed exponentially with a mean at 2.2kcal/Mol. (Forsén & Linse).

Let's check our derivation (and our implementation) of $p_\mathrm{config}$ by comparing it to our direct computation of $p_b$ from §1. Single TF.

def enumerate_configs(TFBSs): return list(map(np.array, itertools.product([0,1], repeat=len(TFBSs))))

def p_configs(TFBSs, TF_conc, cooperativities):

    configs = enumerate_configs(TFBSs)
    weights = []

    for config in configs:

        weights.append(np.exp(log_P_config(config, TFBSs=TFBSs, TF_conc=TF_conc, cooperativities=cooperativities)))

    return list(zip(configs, np.array(weights) / sum(weights)))

p_bound (prev method): 	 0.00921878699097193
p_config (new method): 	 [(array([0]), 0.9907812130090282), (array([1]), 0.00921878699097194)]

With that sanity check, let's now consider the scenario of two transcription factors with cooperative binding, and compute the probabilities of each of the 4 configurations:

Low-Affinity binding

In reality, transcription factors' binding sites are not so discretely present or absent: transcription factors may bind anywhere along the DNA polymer, with an affinity dependent on the interaction surface provided by the sequence of nucleotides at each locus. There are a variety of approaches to model the sequence-to-affinity function for each TF. The simplest is to consider each nucleotide independently, and list the preferences for each nucleotide at each sequential position in a matrix. This type of "mono-nucleotide position weight matrix" (PWM) model is commonly used, and frequently represented by a "sequence logo" plot.

PWMs: Construction

I have curated PWMs from various databases for ~1000 human TFs.

tfdb2 = pd.read_json('../../AChroMap/data/processed/TF.2022.json').fillna(value=np.nan)  ## prefer nan to None

cisbp_pwms = pd.read_pickle('../../tfdb/data/processed/CISBP_2_PWMs.pickle')
humanTFs_pwms = pd.read_pickle('../../tfdb/data/processed/HumanTFs_PWMs.pickle')
jaspar2022_pwms = pd.read_pickle('../../tfdb/data/processed/jaspar2022_PWMs.pickle')
probound_pwms = pd.read_json('../../AChroMap/data/processed/TF_ProBound.json', orient='records').T  # we only get probound as delta G scores, not as PWMs

As an example, consider the Transcription Factor SPI1 / PU.1, for which the following PWMs are registered in the CisBP 2.00 database:

SPI1_pwms = tfdb2.loc[(tfdb2.Name == 'SPI1')]

SPI1_cisbp_pwms = cisbp_pwms.loc[SPI1_pwms.CISBP_2_strict.iat[0]]
SPI1_humanTFs_pwms = humanTFs_pwms.reindex(SPI1_pwms.HumanTFs.iat[0]).dropna()
SPI1_jaspar2022_pwms = jaspar2022_pwms.loc[SPI1_pwms.JASPAR_2022.iat[0]]
SPI1_probound_pwms = probound_pwms.loc[SPI1_pwms.ProBound.iat[0]].pwm

for (name, SPI1_pwm), offset in zip(SPI1_cisbp_pwms.items(), (0,0,2,0,0,0)):
    ax = plot_pwm(SPI1_pwm, figsize=((len(SPI1_pwm)+offset+1)*0.25,1), xtick_offset=offset)
    ax.set_title(name, loc='left')

This representation of this matrix visualizes the proportion of occurrences of a base at a particular position in aligned SPI1 binding sites. Since they look similar, we may be tempted to average them to have a single model of PU.1 binding, however it is considered unwise to do so, as TFs are believed to have multiple binding "modes" which refers explicitly to modes in the distribution of sequence-binding affinities.

These PWM models can be fit from a variety of experiments measuring PU.1 binding. In this case, we're looking at models fit from High-Throughput SELEX data, but other models for PU.1 binding generated from other types of experiments are catalogued at its entry in the HumanTFs database.

PWMs: scoring sequences

If we assume a TF's PWM catalogues its relative binding preferences to every possible sequence of individual bases, we can use that PWM to evaluate the relative probabilities of TF binding to new sequences. We'll define the PWM "score" as the (log) ratio of the probability of the sequence with the PWM against random chance. The probability of a sequence under the PWM is $\prod \mathrm{PWM}[i][\mathrm{dna}[i]]$. The probability of the sequence under a background model is $\prod \mathrm{bg}(\mathrm{dna}[i])$ where $\mathrm{bg}$ describes the frequency of each base in the genome (not quite 1/4 in the human genome). We take the logarithm of the ratio of these two probabilities to produce the log-likelihood ratio, which we call our "score":

$$\mathrm{score}(\mathrm{PWM}, j) = \log(\frac{\prod \mathrm{PWM}[i][\mathrm{dna}[j+i]]}{\prod \mathrm{bg}(\mathrm{dna}[j+i])}) = \sum \log(\frac{ \mathrm{PWM}[i][\mathrm{dna}[j+i]]}{\mathrm{bg}(\mathrm{dna}[j+i])}) $$

A positive $\mathrm{score}(\mathrm{PWM}, j)$ indicates the subsequence starting at position $j$ is likelier under the PWM model than a random sequence from the human genome, and a negative score indicates the subsequence is more likely to be random. Let's visualize a PU.1 scoring matrix:

bg = [0.2955, 0.2045, 0.2045, 0.2955]
pseudocount = 0.0001

def log_odds_pwm(pwm):
    return np.log((pwm+pseudocount)/bg)

pwm = SPI1_cisbp_pwms.iloc[1]
pwm_name = SPI1_cisbp_pwms.index[0]
ax = plot_pwm(log_odds_pwm(pwm), figsize=(4,4))
_ = ax.set_ylabel('score',  weight='bold')

cisbp_score_pwms = cisbp_pwms.apply(log_odds_pwm)
humanTFs_score_pwms = humanTFs_pwms.apply(log_odds_pwm)
jaspar2022_score_pwms = jaspar2022_pwms.apply(log_odds_pwm)

SPI1_cisbp_score_pwms = cisbp_score_pwms.loc[SPI1_pwms.CISBP_2_strict.iat[0]]
SPI1_humanTFs_score_pwms = humanTFs_score_pwms.reindex(SPI1_pwms.HumanTFs.iat[0]).dropna()
SPI1_jaspar2022_score_pwms = jaspar2022_score_pwms.loc[SPI1_pwms.JASPAR_2022.iat[0]]

Our strategy will be to score genomic regions for TF binding, searching for high PWM scores starting at every offset $j$ in the supplied DNA sequence. Let's remind ourselves here that in order to find the full set of motif matches in a supplied DNA sequence, we either need to scan the reference genome and its reverse complement, or scan the positive strand with both the forward and reverse-complement PWMs. We opt for the latter approach, and therefore augment our PWM set with reverse-complement PWMs:

plot_pwm(pwm, figsize=(4,1)).set_title('Forward: '+pwm_name, loc='left')
plot_pwm(reverse_complement_pwm(pwm), figsize=(4,1)).set_title('Reverse: '+pwm_name, loc='left')
None

Since we now have sequence-dependent transcription factor binding models, we will select a sequence which harbors known binding sites for select transcription factors, and see whether the models predict binding of those transcription factors.

As a specific sequence, let's use the SPI1 promoter.

def get_reference_transcript(gene_name):

    hg38_reference_annotation = pd.read_csv('../../AChroMap/data/processed/GRCh38_V29.csv')
    reference_transcript = hg38_reference_annotation[(hg38_reference_annotation.gene_name == gene_name) & (hg38_reference_annotation.canonicity == 0)]
    chrom = reference_transcript.chr.iat[0]
    strand = reference_transcript.strand.iat[0]
    tss = (reference_transcript.txStart if all(reference_transcript.strand == 1) else reference_transcript.txEnd).iat[0]

    return chrom, strand, tss

gene_name = 'SPI1'

chrom, strand, tss = get_reference_transcript(gene_name)
start = tss-400
stop = tss+400

def get_hg38_bases_local(chrom, start, stop):
    seqkit_command = f'seqkit subseq /Users/alex/Documents/AChroMap/data/raw/genome/hg38_fa/{chrom}.fa --region {start}:{stop}'
    dna = subprocess.run(shlex.split(seqkit_command), stdout=subprocess.PIPE).stdout.decode('utf-8').split('\n',1)[1].replace('\n','')
    return dna

SPI1_promoter_dna_sequence = get_hg38_bases_local(chrom, start, stop)
print(SPI1_promoter_dna_sequence)

TTCCAGGGAGGAAACCCTGACTTCCCACTGATAGCAAGCCAGGAGGGCAGTGGGTGGGCTGGCGGGTTCGTGGGCAGGCAGGCAGGCGTCCGAGGGCCACGGGTTGGGCTGGTGGAGGAGTCCCGGTACTCACAGGGGGGACGAGGGGAAACCCTTCCATTTTGCACGCCTGTAACATCCAGCCGGGCTCCGAGTCGGTCAGATCCCCTGCCTCGGTGGGGGCCAATGCAGAGCCCCTCAGGATGGGGTGCCCCGTCAGGGGCTGGACGGTCGTGGGGCGGGTGCAGGGCTCAGGCCTGCCCCCTGAGCTACAGGAGCCCTGGGTGAGCCCCCTCCCTTGACATTGCAGGGCCAGCACAAGTTCCTGATTTTATCGAAGGGCCTGCCGCTGGGAGATAGTCCCCTTGGGGTGACATCACCGCCCCAACCCGTTTGCATAAATCTCTTGCGCTACATACAGGAAGTCTCTGGCCGGCTGGGGCAGGTGGTGCTCAAAGGGCTGGCCTGGGAAGCCATGGGGTCCAGGCCCCCTGCCCAGAGGAAGCTGGGACTGAGAGGGATGACTTTGGGGGCTAAGCTGGGGAGGGAGGATGGGAGGGAGAACGTGTAGCTCTGCCACACCACTGGGAGGCTTTTGCTCTAACCCAACAAATGCCTGCTTCTTTTGAGATCCCTATGTAGCCAACAGTCACCTCATTGGGGTCAGAGCTGGAAGGGGTGGCCTCTTGGGGCCTCCACTTTCTGGAGTCAGCCTTCCTGGGTGAGGGCTCTGATCTAGCAGGCTATCAGGCCTGGCTCTTC

SPI1 promotes its own transcription, by binding to its own promoter. We can scan the SPI1 promoter for significant SPI1 PWM scores to locate the binding site.

base_index = {'a':0,'c':1,'g':2,'t':3,'A':0,'C':1,'G':2,'T':3}

def scan_sequence(pwm, dna):
    scores = []

    for i in range(len(dna)):
        score = 0
        for j, row in enumerate(pwm):
            if i+j < len(dna):
                score += row[base_index[dna[i+j]]]

        scores.append(score)

    return np.array(scores)

SPI1_PWM_scores = pd.DataFrame(SPI1_cisbp_score_pwms.apply(scan_sequence, args=(SPI1_promoter_dna_sequence,)).values.tolist(), index=SPI1_cisbp_score_pwms.index).T
SPI1_PWM_scores.index += start

positive_SPI1_PWM_scores = pd.DataFrame(np.where(SPI1_PWM_scores > 0, SPI1_PWM_scores, np.nan), index=SPI1_PWM_scores.index, columns=SPI1_PWM_scores.columns)

def genomic_xticks(ax, chrom=None):
    xlims = ax.get_xlim()
    xtick_vals = ax.get_xticks()
    ax.set_xticks(xtick_vals)
    ax.set_xticklabels(['{:.0f}'.format(x) for x in xtick_vals])
    ax.set_xlim(xlims)
    if chrom: ax.set_xlabel(chrom)

ax = SPI1_PWM_scores.plot(lw=0.1, xlim=(SPI1_PWM_scores.index.min(),SPI1_PWM_scores.index.max()), legend=False)
ax = positive_SPI1_PWM_scores.plot(style=".", ax=ax)
ax.set_ylabel('score')
genomic_xticks(ax)

def plot_tss(ax, tss, strand, axvline=False):
    arrow_direction = 8 if strand == -1 else 9
    ax.plot(tss, 1, lw=0, markersize=10, c='deepskyblue', marker=arrow_direction)
    ax.text(tss+1,0,gene_name)
    if axvline: ax.axvline(tss, c='deepskyblue', lw=0.5)

plot_tss(ax, tss, strand)
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-07-19T15:43:30.560584 image/svg+xml Matplotlib v3.5.2, https://matplotlib.org/

Now we'd like to score every PWM against every offset in the entire reference human genome. In order to accomplish that, we'll use MOODS, which implements a faster scanning algorithm in C++. We'll use this script to score the human reference genome with our PWMs.

PWMs: Score cutoffs

Once we have scanned a sequence of genomic DNA, scoring the PWM at every offset, we need to establish which of those scores is "significant" enough that we would predict the existence of a transcription factor binding site at that position in the genome. We can imagine two approaches to distinguish "significant" motif matches for each PWM. Thresholding on a particular score corresponds to predicting the TF is bound to sites which are $e^{\mathrm{score}}$ more likely under the PWM than random. A score cutoff of 6.91 is 1000x more likely under the PWM than random, for every PWM.

Contrarily, if you wanted to choose the 0.1% top scores for each PWM, each PWM would have a unique score cutoff, as each cutoff would depend on the distribution of scores specified by the PWM. At first this may seem surprising, so we'll explore it empirically for two PWMs:

pwm_names = ['M07944_2.00','M03355_2.00']
pwms = cisbp_pwms.loc[pwm_names]
score_pwms = cisbp_score_pwms.loc[pwm_names]
for name, pwm in pwms.items(): plot_pwm(pwm, figsize=(4,1)).set_title(name, loc='left')

def sample_scores_from_pwm(pwm, sample_size=int(1e6)):
    scores = np.zeros(sample_size)
    for i in range(len(pwm)):
        scores += np.random.choice(pwm[i], sample_size, p=bg)
    return pd.Series(scores)

score_samples = score_pwms.apply(sample_scores_from_pwm).T
ax = score_samples.plot.hist(bins=100, alpha=0.5)
ax.set(xlabel='score')
fig_style_2(ax)

Clearly we can see these two PWMs define different score distributions for random sequences. Let's see what probabilities the highest scores correspond to for each of these PWMs, using MOODS' score cutoff method:

def score_thresholds(score_pwm):
    min_significance, max_significance = -2, -15
    significance_range = min_significance - max_significance
    pvals = np.logspace(min_significance, max_significance, significance_range*2+1)
    score_thresholds = [MOODS.tools.threshold_from_p(score_pwm.T.tolist(), bg, p) for p in pvals]
    return pd.Series(score_thresholds, index=pvals)

score_quantiles = score_pwms.apply(score_thresholds).T.rename_axis('p').reset_index()

fig, ax = plt.subplots()

cols = score_quantiles.columns[1:]
for col in cols:
    score_quantiles.plot(x=col, y='p', logy=True, legend=False, ax=ax)

ax.set(ylabel='p_value', xlabel='score')
ax.grid(True, ls=':')
for side in ["right","top"]: ax.spines[side].set_visible(False)
ax.legend(cols)
ax.axhline(1e-3, c='red')
ax.text(0,2e-3, 'p=0.001')
ax.plot(score_quantiles.iloc[2][['M07944_2.00','M03355_2.00']],(1e-3, 1e-3), marker='o', lw=0, c='red')
None

For each motif, we can compute which score corresponds to each p-value cutoff, and then estimate p-values for scores of motif matches discovered during scanning using an interpolation. We'll use a generic spline as an interpolation function.

# cisbp_score_quantiles.to_csv('../data/equilibrium_TFs/cisbp_score_quantiles.csv', index=False)
cisbp_score_quantiles = pd.read_csv('../notebook_data/equilibrium_TFs/cisbp_score_quantiles.csv')

def create_score_to_pval_interpolant(motif_name):
    quantiles = cisbp_score_quantiles[[motif_name, 'p']]
    quantiles = quantiles[quantiles[motif_name] >= quantiles[motif_name].cummax()].drop_duplicates(subset=[motif_name], keep='first')  # guarantee strict monotonic increasing
    score_to_pval_interpolation = interpolate.InterpolatedUnivariateSpline(quantiles[motif_name], quantiles['p'], ext=3)
    return score_to_pval_interpolation

#     create_score_to_pval_interpolant(motif_name)
#     tfbs.loc[tfbs.name == motif_name, 'p'] = tfbs.loc[tfbs.name == motif_name, 'score'].apply(score_to_pval_interpolation)

PWMs: Other formalisms

Unfortunately, the PWM formalism we have introduced misses the biophysical interpretation we have carried throughout our analysis thus far. We can coax a biophysical interpretation from these PWMs as follows (following the approach from Foat, Morozov, Bussemaker, 2006):

If we consider the nucleotides independently, then the ratio of equilibrium binding to a given nucleotide $j$ versus the highest-affinity nucleotide $\mathrm{best}$ at each position is related to the difference in energies of binding:

$$ \frac{[TF:N_j]}{[TF:N_{best}]} = \frac{K_{a_j}}{K_{a_{best}}} = \exp \{\Delta G_j - \Delta G_{best} \} $$

With this expression, we can compute the energy of binding of a TF to a sequence relative to that TF's energy of binding to its best sequence of nucleotides. However, we need to know an absolute energy of binding to anchor this relative measure.

cisbp_energy_pwms = cisbp_pwms.apply(make_energy_pwm)
humanTFs_energy_pwms = humanTFs_pwms.apply(make_energy_pwm)
jaspar2022_energy_pwms = jaspar2022_pwms.apply(make_energy_pwm)

We can visualize this "energy PWM" as well. Visualizing energies of particular bases relative to SPI1's best sequence does not produce a visual representation that's easy to reason about:

SPI1_cisbp_energy_pwms = cisbp_energy_pwms.loc[SPI1_cisbp_pwms.index]
SPI1_cisbp_energy_pwm = SPI1_cisbp_energy_pwms[0]
ax = plot_pwm(SPI1_cisbp_energy_pwm)

We can rescale this matrix to the average sequence:

ax = plot_pwm(SPI1_cisbp_energy_pwm - np.expand_dims(SPI1_cisbp_energy_pwm.mean(axis=1), axis=1))

However, the ideal rescaling of this PWM would indicate whether each nucleotide contributes positively or negatively towards the energy of binding. After all, SPI1 likely doesn't bind the "average" sequence -- which is to say it's off rate is higher than it's on rate.

We chose SPI1 / PU.1 as our prototype in part because its absolute binding energies to various sequences have been measured, allowing us to anchor our relative energies PWM model. Pham et al measured PU.1 binding to a variety of high-affinity sequences by microscale thermophoresis and recorded a $k_d$ of 156nm for the best one.

SPI1_best_nanomolar_kd = 156
SPI1_best_ΔG = kcal_ΔG_from_nanomolar_kd(SPI1_best_nanomolar_kd)
SPI1_best_ΔG

9.648885505793956

However, the question remains of how to allocate those ~9.6 kcal/mol across the 14 bases of the SPI1 motif. The naive option is to uniformly spread the binding energy across the bases (9.6/14). Instead, we'll distribute it proportionally to the variance at each position to capture the intuition that the flanking nucleotides contribute less.

SPI1_cisbp_energy_pwm_scaled = make_abs_pwm(SPI1_cisbp_energy_pwm, SPI1_best_ΔG)
ax = plot_pwm(SPI1_cisbp_energy_pwm_scaled)

At last we have a visual representation of of a mono-nucleotide sequence preference model which captures our intuitions about the energetics of TF binding.

For a restricted set of TFs with high-quality HT-SELEX data, Rube et al produced relative energy PWMs with higher accuracy in the low-affinity regime. SPI1 is among that privileged set, so we'll take a look at that PWM for comparison.

SPI1_probound_pwms_scaled = SPI1_probound_pwms.apply(lambda pwm: make_abs_pwm(pwm, SPI1_best_ΔG))
ax = plot_pwm(SPI1_probound_pwms_scaled.iloc[2])

PWMs: Evaluation

To what extent do these PWM models predict real transcription factor binding? In order to evaluate these models, we would like to compare their predictions with ground truth measurements of transcription factor occupancy.

  • TF occupancy can be estimated with ChIP. Has been estimated in K562 (and HepG2)

Limitations:

  • We only have PWMs for 1000 TFs,
  • We only have ChIP for 600 TFs

What's the intersection?

all_chip_able_targets = pd.read_csv('/Users/alex/Documents/AChroMap/data/raw/ENCODE/k562_chip/metadata.cleaned.tsv', sep='\t').Target

pwm_cols = ['CISBP_2_strict','CISBP_2_intermediate','CISBP_2_tolerant','HumanTFs','JASPAR_2022','ProBound']
TF_coverage = {pwm_db: tfdb2[tfdb2.set_index('Name')[pwm_db].notnull().values].Name for pwm_db in pwm_cols}

fig, axs = plt.subplots(2, len(pwm_cols)//2)
for pwm_db, ax in zip(pwm_cols, axs.flatten()):
    colors = ['dodgerblue', 'seagreen', 'lightsteelblue']
    venn_obj = venn3((set(TF_coverage[pwm_db]), set(all_chip_able_targets), set(tfdb2.Name)),
                     (f'{pwm_db} ({len(set(TF_coverage[pwm_db]))})', f'ChIP in K562 ({len(set(all_chip_able_targets))})', f'DNA-binding TF ({len(set(tfdb2.Name))})'),
                     colors, alpha=0.6, ax=ax)
    for text in venn_obj.set_labels: text.set_fontsize(8)
    for text in [x for x in venn_obj.subset_labels if x]: text.set_fontsize(6)
    venn_obj.set_labels[0].set_fontweight(700)
    venn_obj.get_patch_by_id('111').set_edgecolor('green')

TFs_to_evaluate = set(all_chip_able_targets) & set(TF_coverage['CISBP_2_strict'])

pd.Series(list(TFs_to_evaluate)).to_csv('../notebook_data/TFs_to_evaluate.csv', index=False, header=False)

In ATAC OCRs

  • Justify only looking inside ATAC peaks / OCRs.

explain motivation: want to look in all ATAC regions for PWMs and chip signal in k562s

narrowpeak_cols = ['chrom','chromStart','chromEnd','name','score','strand','signalValue','pValue','qValue','summit']

ATAC_peaks_files = ['/Users/alex/Documents/AChroMap/data/raw/ENCODE/k562_atac/downloads/ENCFF333TAT.bed',
                    '/Users/alex/Documents/AChroMap/data/raw/ENCODE/k562_atac/downloads/ENCFF558BLC.bed']

ATAC_signal_files = ['/Users/alex/Documents/AChroMap/data/raw/ENCODE/k562_atac/downloads/ENCFF357GNC.bigWig',
                     '/Users/alex/Documents/AChroMap/data/raw/ENCODE/k562_atac/downloads/ENCFF600FDO.bigWig']

def filter_overlapping_peaks_for_max_signalValue(filepath, fraction_either=0.8):

    narrowpeaks_unfiltered = pd.read_csv(f'{filepath}', sep='\t', na_values=['NAN'], header=None, names=narrowpeak_cols)

    bedops_command = f"bedops -u {filepath} | cut -f1-4,7 | bedmap --fraction-either {fraction_either} --max-element - | sort-bed --unique - > {filepath}.tmp"
    os.system(bedops_command)
    narrowPeaks_filtered_peak_names = pd.read_csv(f'{filepath}.tmp', sep='\t', na_values=['NAN'], header=None, names=narrowpeak_cols[:5]).name
    os.remove(f'{filepath}.tmp')

    return narrowpeaks_unfiltered[narrowpeaks_unfiltered.name.isin(narrowPeaks_filtered_peak_names)]

K562_ATAC_peaks_1_filtered = filter_overlapping_peaks_for_max_signalValue(ATAC_peaks_files[0])
K562_ATAC_peaks_2_filtered = filter_overlapping_peaks_for_max_signalValue(ATAC_peaks_files[1])

K562_ATAC_peaks_1_filtered['signalValue_percentile'] = K562_ATAC_peaks_1_filtered.signalValue.rank(pct=True)
K562_ATAC_peaks_2_filtered['signalValue_percentile'] = K562_ATAC_peaks_2_filtered.signalValue.rank(pct=True)

def get_overlapping_peaks(peaks_bed, chrom, start, stop):

    intervals = pd.IntervalIndex.from_arrays(peaks_bed.chromStart, peaks_bed.chromEnd)

    return peaks_bed[(peaks_bed.chrom == chrom) & intervals.overlaps(pd.Interval(start, stop))]

atac_peak_at_SPI1_promoter_1 = get_overlapping_peaks(K562_ATAC_peaks_1_filtered, chrom, start, stop)
atac_peak_at_SPI1_promoter_2 = get_overlapping_peaks(K562_ATAC_peaks_2_filtered, chrom, start, stop)

def get_bigwig_values(url, chrom, start, stop):
    with pyBigWig.open(url) as bw:
        values = bw.values(chrom, start, stop)
    return values

ATAC_bigwig_1 = get_bigwig_values(ATAC_signal_files[0], chrom, earliest_start, latest_end)
ATAC_bigwig_2 = get_bigwig_values(ATAC_signal_files[1], chrom, earliest_start, latest_end)

ATAC_bigwig = pd.DataFrame([ATAC_bigwig_1, ATAC_bigwig_2], columns=range(earliest_start, latest_end), index=['ATAC_1', 'ATAC_2']).T

ax = ATAC_bigwig.plot.line()
plot_tss(ax, tss, strand)
genomic_xticks(ax)

from this we conclude that the first set of ATAC peaks is a richer dataset, and proceed with those

ATAC_OCRs_to_evaluate_PWMs_in = K562_ATAC_peaks_1_filtered[K562_ATAC_peaks_1_filtered.score == 1000]

ATAC_OCRs_to_evaluate_PWMs_in.to_csv('../notebook_data/equilibrium_TFs/K562_ATAC_peaks_filtered.bed', sep='\t', index=False)

Get DGF 

motif_cluster_and_gene = pd.read_csv('/Users/alex/Documents/AChroMap/data/raw/Vierstra_supp/motif_cluster_and_gene.csv', index_col=0)
motif_clusters_to_TFs = pd.read_csv('/Users/alex/Documents/AChroMap/data/raw/Vierstra_supp/motif_clusters_to_TFs.csv', index_col=0)
motif_clusters_to_TFs = motif_clusters_to_TFs.set_index('Name')['gene'].str.split(';')

DGF_footprint_index = pd.read_csv('/Users/alex/Documents/AChroMap/data/raw/Vierstra_supp/consensus_index_matrix_full_hg38.index.csv', index_col=0, header=None).index
DGF_samples_index = pd.read_csv('/Users/alex/Documents/AChroMap/data/raw/Vierstra_supp/consensus_index_matrix_full_hg38.columns.csv', index_col=0, header=None).index
K562_DGF_samples = DGF_samples_index[DGF_samples_index.str.startswith('K562')].tolist()

def get_k562_DGF(chrom, start, end):

    dgf_path = '/Users/alex/Documents/AChroMap/data/raw/Vierstra_supp/consensus_footprints_and_collapsed_motifs_hg38.bed.gz'
    tabix_coords = chrom+':'+str(start)+'-'+str(end)
    cols = ['contig','start','stop','identifier','mean_signal','num_samples','num_fps','width','summit_pos','core_start','core_end','motif_clusters']
    tabix_command = f'tabix {dgf_path} {tabix_coords}'
    footprints_contained_in_region = read_shell(shlex.split(tabix_command), sep='\t', header=None, names=cols, dtype={'index_id': str})

    footprints_contained_in_region['TFs'] = footprints_contained_in_region.motif_clusters.str.split(';').apply(lambda l: [motif_clusters_to_TFs[motif_cluster] for motif_cluster in l] if (type(l) == list) else [])
    first_dgf_index = DGF_footprint_index.get_loc(footprints_contained_in_region.identifier.iloc[0])
    last_dgf_index = DGF_footprint_index.get_loc(footprints_contained_in_region.identifier.iloc[-1])+1

    footprint_posterior = pd.read_hdf('/Users/alex/Documents/AChroMap/data/raw/Vierstra_supp/consensus_index_matrix_full_hg38.hdf', key='consensus_index_matrix_full_hg38',
                                      start=first_dgf_index, stop=last_dgf_index, columns=K562_DGF_samples)

    footprint_binary = pd.read_hdf('/Users/alex/Documents/AChroMap/data/raw/Vierstra_supp/consensus_index_matrix_binary_hg38.hdf', key='consensus_index_matrix_full_hg38',
                                   start=first_dgf_index, stop=last_dgf_index, columns=K562_DGF_samples)

    k562_dgf = footprints_contained_in_region.merge(footprint_posterior, how='left', left_on='identifier', right_index=True)
    k562_dgf = k562_dgf.merge(footprint_binary, how='left', left_on='identifier', right_index=True, suffixes=['_posterior','_binary'])

    return k562_dgf

k562_dgf = get_k562_DGF(chrom, start, stop)

k562_dgf.plot.scatter(x='K562-DS15363_posterior', y='K562-DS16924_posterior', figsize=(4,4), logx=True, logy=True)

<AxesSubplot:xlabel='K562-DS15363_posterior', ylabel='K562-DS16924_posterior'>
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-07-19T14:24:49.694426 image/svg+xml Matplotlib v3.5.2, https://matplotlib.org/

Load K562 RNA 

ENCODE_RNA_metadata = pd.read_csv('../../AChroMap/data/raw/ENCODE/rna/metadata.tsv', sep='\t')
ENCODE_RNA_metadata_cleaned = pd.read_csv('../../AChroMap/data/raw/ENCODE/rna/metadata.clean.typed.csv')
ENCODE_polyA_metadata = pd.read_csv('../../AChroMap/data/raw/ENCODE/polyA/metadata.tsv', sep='\t')
ENCODE_polyA_metadata_cleaned = pd.read_csv('../../AChroMap/data/raw/ENCODE/polyA/metadata.clean.typed.csv')

rna_counts = pd.read_csv('../../AChroMap/data/raw/ENCODE/rna/RSEM_gene/RSEM_gene.counts_matrix.wellexpressed_deseq.csv', index_col=0)
polyA_counts = pd.read_csv('../../AChroMap/data/raw/ENCODE/polyA/RSEM_gene/RSEM_gene.counts_matrix.wellexpressed_deseq.csv', index_col=0)

K562_reference_polyA = pd.read_csv('/Users/alex/Desktop/ENCFF472HFI.tsv', sep='\t')
K562_reference_polyA = K562_reference_polyA[K562_reference_polyA.gene_id.str.startswith('ENSG')]
K562_reference_polyA['gene_id'] = K562_reference_polyA.gene_id.str.split('.').str[0]
K562_reference_polyA = K562_reference_polyA.set_index('gene_id')

K562_RNA_metadata = ENCODE_RNA_metadata[ENCODE_RNA_metadata['Biosample term name'] == 'K562']
K562_RNA_metadata_cleaned = ENCODE_RNA_metadata_cleaned[ENCODE_RNA_metadata_cleaned['Biosample term name'] == 'K562']
K562_polyA_metadata = ENCODE_polyA_metadata[ENCODE_polyA_metadata['Biosample term name'] == 'K562']
K562_polyA_metadata_cleaned = ENCODE_polyA_metadata_cleaned[ENCODE_polyA_metadata_cleaned['Biosample term name'] == 'K562']

K562_RNA_metadata_cleaned = K562_RNA_metadata_cleaned[K562_RNA_metadata_cleaned.type == 'RSEM_gene']
K562_polyA_metadata_cleaned = K562_polyA_metadata_cleaned[K562_polyA_metadata_cleaned.type == 'RSEM_gene']

K562_RNA_metadata = K562_RNA_metadata[K562_RNA_metadata['File accession'].isin(K562_RNA_metadata_cleaned.file)]
K562_polyA_metadata = K562_polyA_metadata[K562_polyA_metadata['File accession'].isin(K562_polyA_metadata_cleaned.file)]

K562_RNA_metadata = K562_RNA_metadata[K562_RNA_metadata['Audit WARNING'].isnull() & K562_RNA_metadata['Audit ERROR'].isnull() & K562_RNA_metadata['Audit NOT_COMPLIANT'].isnull()]
K562_polyA_metadata = K562_polyA_metadata[K562_polyA_metadata['Audit WARNING'].isnull() & K562_polyA_metadata['Audit ERROR'].isnull() & K562_polyA_metadata['Audit NOT_COMPLIANT'].isnull()]

K562_RNA_metadata_cleaned = K562_RNA_metadata_cleaned[K562_RNA_metadata_cleaned.file.isin(K562_RNA_metadata['File accession'])]
K562_polyA_metadata_cleaned = K562_polyA_metadata_cleaned[K562_polyA_metadata_cleaned.file.isin(K562_polyA_metadata['File accession'])]

K562_rna_counts = rna_counts.loc[rna_counts.index.str.startswith('ENSG'), rna_counts.columns.isin(K562_RNA_metadata['File accession'])]
K562_polyA_counts = polyA_counts.loc[polyA_counts.index.str.startswith('ENSG'), polyA_counts.columns.isin(K562_polyA_metadata['File accession'])]

K562_rna_counts.index = K562_rna_counts.index.str.split('.').str[0]
K562_polyA_counts.index = K562_polyA_counts.index.str.split('.').str[0]

best_polyA_files = K562_polyA_metadata_cleaned[K562_polyA_metadata_cleaned.experiment.isin(['ENCSR000AEP','ENCSR000AEQ'])].file.tolist()
best_rna_files = K562_RNA_metadata_cleaned[K562_RNA_metadata_cleaned.experiment.isin(['ENCSR792OIJ'])].file.tolist()

def series_to_distplot(s):
    sns.kdeplot(data=s)
    sns.rugplot(data=s, height=.1)

rna_counts_sum = K562_rna_counts.sum()
series_to_distplot(rna_counts_sum)
ax = plt.gca()
for x in rna_counts_sum[best_rna_files].values:
    ax.axvline(x, c='r', lw=0.3)

plt.subplots()
polyA_counts_sum = K562_polyA_counts.sum()
series_to_distplot(polyA_counts_sum)
ax = plt.gca()
for x in polyA_counts_sum[best_polyA_files].values:
    ax.axvline(x, c='r', lw=0.3)
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-07-20T11:59:09.721224 image/svg+xml Matplotlib v3.5.2, https://matplotlib.org/
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-07-20T11:59:09.792664 image/svg+xml Matplotlib v3.5.2, https://matplotlib.org/ 
mRNAs_per_cell = 1e5  # citation follows
RNAs_per_cell = 4e5  # this one is a guess

K562_polyA_TPC = K562_polyA_counts / K562_polyA_counts.sum() * mRNAs_per_cell
K562_rna_TPC = K562_rna_counts / K562_rna_counts.sum() * RNAs_per_cell

(Marinov... Wold, 2014)

K562_RNA_TF_TPC = K562_rna_TPC[K562_rna_TPC.index.isin(tfdb2.ID)]
K562_polyA_TF_TPC = K562_polyA_TPC[K562_polyA_TPC.index.isin(tfdb2.ID)]

K562_polyA_TF_TPC.index = tfdb2.set_index('ID').reindex(K562_polyA_TF_TPC.index).Name.values

plt.rcParams['figure.figsize'] = [17, 5]
g = sns.stripplot(data=K562_polyA_TF_TPC.iloc[:100].T, alpha=0.4)
plt.xticks(rotation=-90)

g = sns.stripplot(data=K562_polyA_TF_TPC.iloc[:100][best_polyA_files].T, color='black', s=2, marker="D")
# also plot the median

None
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-07-20T12:38:35.153413 image/svg+xml Matplotlib v3.5.2, https://matplotlib.org/ 
 

K562_polyA_TF_TPC.to_csv('../notebook_data/equilibrium_TFs/K562_TF_RNA.csv')

RNA_to_protein_linear_scaling_factor = 9800

K562_TF_copy_number = K562_polyA_TF_TPC.replace(0, np.nan).median(axis=1).replace(np.nan, 0) * RNA_to_protein_linear_scaling_factor

(Li,... Biggin, 2014)

plt.rcParams['figure.figsize'] = [12, 5]

K562_TF_copy_number.plot.hist(bins=100, logy=True)

<AxesSubplot:ylabel='Frequency'>
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-07-20T13:50:09.911002 image/svg+xml Matplotlib v3.5.2, https://matplotlib.org/ 
K562_TF_nanomolar_conc = K562_TF_copy_number.apply(copy_number_and_cubic_micron_volume_to_molar_concentration, args=(K562_volume_micron_cubed,)) * 1e9

K562_TF_RNA_concise = pd.concat((tfdb2.set_index('Name').ID.reindex(K562_TF_copy_number.index),
                                 K562_TF_copy_number.rename('TF_protein_copy_number_estimate'),
                                 K562_TF_nanomolar_conc.rename('nanomolar_conc')), axis=1)

K562_TF_RNA_concise = K562_TF_RNA_concise.rename_axis('Name').reset_index().set_index('ID')
K562_TF_RNA_concise = K562_TF_RNA_concise.sort_values('TF_protein_copy_number_estimate', ascending=False).drop_duplicates(subset=['Name'])

K562_TF_RNA_concise.to_csv('../notebook_data/equilibrium_TFs/K562_TF_RNA_concise.csv')

Evaluation of PWMs at the SPI1 promoter

We've just generated a list of OCRs we want to evaluate PWMs for, but let's start with our single example to determine how to evaluate PWMs vs ChIP.

# chrom, strand, tss = get_reference_transcript(gene_name)
# start = tss-400
# stop = tss+400
# K562_TF_RNA = pd.read_csv('../notebook_data/equilibrium_TFs/K562_TF_RNA.csv', index_col=0)
Get TF ChIP Peaks at SPI1 Promoter 
def get_K562_TF_ChIP_peaks(chrom, start, end):

    k562_merged_ChIP_path = '/Users/alex/Documents/AChroMap/data/raw/ENCODE/k562_chip/K562_ChIP_merged.bed.gz'
    tabix_coords = chrom+':'+str(start)+'-'+str(end)
    tabix_command = f'tabix {k562_merged_ChIP_path} {tabix_coords}'
    peaks_contained_in_region = read_shell(shlex.split(tabix_command), sep='\t', header=None, names=['chrom','chromStart','chromEnd','name','signalValue'])

    return peaks_contained_in_region

K562_SPI1_TF_chip_peaks = get_K562_TF_ChIP_peaks(chrom, start, stop)
peaks_df = K562_SPI1_TF_chip_peaks[K562_SPI1_TF_chip_peaks.name.isin(TFs_to_evaluate)]
Get TF ChIP signal over background at SPI1 promoter 
SPI1_promoter_TF_ChIP_signal = pd.read_csv('../notebook_data/equilibrium_TFs/SPI1_promoter_TF_ChIP_signal.csv', index_col=0)
SPI1_promoter_TF_ChIP_signal = SPI1_promoter_TF_ChIP_signal.clip(lower=1).replace(1, np.nan)
signal_df = SPI1_promoter_TF_ChIP_signal.loc[:, SPI1_promoter_TF_ChIP_signal.columns.str.split('_').str[0].isin(TFs_to_evaluate)]
signal_df = signal_df[signal_df.max(axis=0).sort_values(ascending=False).index.values]
Get ATAC signal over background at SPI1 promoter 
SPI1_promoter_TF_ATAC_signal = pd.Series(get_bigwig_values(ATAC_signal_files[0], chrom, start, stop), index=range(start, stop), name='ATAC_signal')
SPI1_promoter_TF_ATAC_signal = SPI1_promoter_TF_ATAC_signal.clip(lower=1).replace(1, np.nan)
Get PWM predictions at SPI1 promoter 
SPI1_key = f'{chrom}/{atac_peak_at_SPI1_promoter_1.chromStart.iat[0]}-{atac_peak_at_SPI1_promoter_1.chromEnd.iat[0]}'
SPI1_cisbp_tfbs = pd.read_hdf('../../AChroMap/scripts/cisbp2_vs_chip.hdf', SPI1_key)
SPI1_cisbp_tfbs = SPI1_cisbp_tfbs[(SPI1_cisbp_tfbs.start > start) & (SPI1_cisbp_tfbs.end < stop)]
SPI1_cisbp_tfbs['p'] = SPI1_cisbp_tfbs.p.clip(lower=1e-10)
SPI1_cisbp_tfbs['log10p'] = -np.log10(SPI1_cisbp_tfbs.p)
3D Ridgeline 
signal_df = SPI1_promoter_TF_ChIP_signal.loc[:, SPI1_promoter_TF_ChIP_signal.columns.str.split('_').str[0].isin(TFs_to_evaluate)]
TF_order = signal_df.max(axis=0).sort_values().index.str.split('_').str[0].drop_duplicates(keep='first')
column_order = pd.Series({col: (TF_order.get_loc(col.split('_')[0]), int(col.split('_')[1])) for col in signal_df.columns}).sort_values().index
signal_df = signal_df[column_order]

signal_df_20 = signal_df.iloc[:, -20:]

columns_to_TF = signal_df_20.columns.to_frame()[0].str.split('_').str[0].rename('TF').rename_axis('track').reset_index().reset_index().set_index('track')
TF_to_columns = columns_to_TF.reset_index().set_index('TF')
plotted_TFs = set(TF_to_columns.index)

genome_linspace = np.transpose(np.tile(signal_df_20.index.values, (len(signal_df_20.T), 1)))
print('genome_linspace:', genome_linspace.shape)
signal_heights = signal_df_20.values
print('signal_heights:\t', signal_heights.shape)
TF_y_index = np.linspace(0,len(signal_df_20.columns)-1,len(signal_df_20.columns))
print('TF_y_index: \t', TF_y_index.shape)

genome_linspace: (800, 20)
signal_heights:	 (800, 20)
TF_y_index: 	 (20,)

signal_verts = []
for y_i in range(len(TF_y_index)):
    # zero at the beginning and the end to get a nice flat bottom on the polygons
    xs = np.concatenate([[genome_linspace[0,y_i]], genome_linspace[:,y_i], [genome_linspace[-1,y_i]]])
    ys = np.concatenate([[0],signal_heights[:,y_i],[0]])
    signal_verts.append(list(zip(xs, ys)))

colors = LinearSegmentedColormap('colormap', cm.jet._segmentdata.copy(), 20)
colors = [colors(i) for i in range(20)]

peak_verts = []
peak_offsets = []

for col, d in columns_to_TF.iterrows():
    for i, peak in peaks_df[peaks_df['name'] == d.TF].iterrows():
        xs = [peak.chromStart, peak.chromStart, peak.chromEnd, peak.chromEnd]
        ys = [0, peak.signalValue/20, peak.signalValue/20, 0]
        peak_verts.append(list(zip(xs, ys)))
        peak_offsets.append(d['index'])

tfbs_verts = []
tfbs_offsets = []

for col, d in columns_to_TF.iterrows():
    for i, tfbs in SPI1_cisbp_tfbs[SPI1_cisbp_tfbs['TFs'] == d.TF].iterrows():
        xs = [tfbs.start, tfbs.start, tfbs.end, tfbs.end]
        ys = [0, tfbs.score/50, tfbs.score/50, 0]
        tfbs_verts.append(list(zip(xs, ys)))
        tfbs_offsets.append(d['index'])

plt.rcParams['figure.figsize'] = [10, len(signal_df.columns)]

fig = plt.figure()
ax = fig.add_subplot(111, projection='3d')

signal_poly = PolyCollection(signal_verts, facecolors=colors)
signal_poly.set_alpha(0.7)
ax.add_collection3d(signal_poly, zs=TF_y_index, zdir='y')

peak_poly = PolyCollection(peak_verts, edgecolors=(0,0,0,0.3), facecolors=(0,0,0,0))
# peak_poly.set_alpha(0.4)
ax.add_collection3d(peak_poly, zs=peak_offsets, zdir='y')

tfbs_poly = PolyCollection(tfbs_verts)
tfbs_poly.set_alpha(1)
ax.add_collection3d(tfbs_poly, zs=tfbs_offsets, zdir='y')


ax.set_xlim3d(genome_linspace.min(), genome_linspace.max())
ax.set_xlabel(chrom)
ax.set_ylim3d(TF_y_index.min(), TF_y_index.max())
ax.set_ylabel('TF')
ax.set_zlim3d(signal_heights.min(), signal_heights.max())
ax.set_zlabel('signal fold change over background')

xtick_vals = ax.get_xticks()
ax.set_xticks(xtick_vals)
ax.set_xticklabels(['{:.0f}'.format(x) for x in xtick_vals])

ax.set_yticks(TF_y_index)
ax.set_yticklabels(signal_df.columns)


ax.xaxis.pane.fill = False
ax.yaxis.pane.fill = False
ax.zaxis.pane.fill = False
ax.xaxis.pane.set_edgecolor('w')
ax.yaxis.pane.set_edgecolor('w')
ax.zaxis.pane.set_edgecolor('w')
ax.grid(False)


ax.get_proj = lambda: np.dot(Axes3D.get_proj(ax), np.diag([1.0, 1.5, 1.0, 1.0]))


plt.show()

---------------------------------------------------------------------------
ValueError                                Traceback (most recent call last)
Input In [156], in <cell line: 23>()
     21 ax.set_ylim3d(TF_y_index.min(), TF_y_index.max())
     22 ax.set_ylabel('TF')
---> 23 ax.set_zlim3d(signal_heights.min(), signal_heights.max())
     24 ax.set_zlabel('signal fold change over background')
     26 xtick_vals = ax.get_xticks()

File ~/miniconda3/envs/py39/lib/python3.9/site-packages/mpl_toolkits/mplot3d/axes3d.py:865, in Axes3D.set_zlim3d(self, bottom, top, emit, auto, zmin, zmax)
    862     top = zmax
    864 self._process_unit_info([("z", (bottom, top))], convert=False)
--> 865 bottom = self._validate_converted_limits(bottom, self.convert_zunits)
    866 top = self._validate_converted_limits(top, self.convert_zunits)
    868 old_bottom, old_top = self.get_zlim()

File ~/miniconda3/envs/py39/lib/python3.9/site-packages/matplotlib/axes/_base.py:3614, in _AxesBase._validate_converted_limits(self, limit, convert)
   3611 converted_limit = convert(limit)
   3612 if (isinstance(converted_limit, Real)
   3613         and not np.isfinite(converted_limit)):
-> 3614     raise ValueError("Axis limits cannot be NaN or Inf")
   3615 return converted_limit

ValueError: Axis limits cannot be NaN or Inf
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-07-13T10:58:49.045547 image/svg+xml Matplotlib v3.5.2, https://matplotlib.org/
2D Ridgeline 
import matplotlib.style as mplstyle
mplstyle.use('fast')

def style_ax(ax):
    ax.spines['top'].set_visible(False)
    ax.spines['right'].set_visible(False)
    ax.spines['bottom'].set_visible(False)
    ax.patch.set_alpha(0)

from matplotlib.ticker import Locator


class MinorSymLogLocator(Locator):
    """
    Dynamically find minor tick positions based on the positions of
    major ticks for a symlog scaling.
    """
    def __init__(self, linthresh):
        """
        Ticks will be placed between the major ticks.
        The placement is linear for x between -linthresh and linthresh,
        otherwise its logarithmically
        """
        self.linthresh = linthresh

    def __call__(self):
        'Return the locations of the ticks'
        majorlocs = self.axis.get_majorticklocs()

        # iterate through minor locs
        minorlocs = []

        # handle the lowest part
        for i in range(1, len(majorlocs)):
            majorstep = majorlocs[i] - majorlocs[i-1]
            if abs(majorlocs[i-1] + majorstep/2) < self.linthresh:
                ndivs = 10
            else:
                ndivs = 9
            minorstep = majorstep / ndivs
            locs = np.arange(majorlocs[i-1], majorlocs[i], minorstep)[1:]
            minorlocs.extend(locs)

        return self.raise_if_exceeds(np.array(minorlocs))

    def tick_values(self, vmin, vmax):
        raise NotImplementedError('Cannot get tick locations for a '
                                  '%s type.' % type(self))

def style_stripplot(ax):

    ax.set_xscale('symlog', linthresh=2)
    ax.get_xaxis().set_major_formatter(matplotlib.ticker.ScalarFormatter())
    # ax.xaxis.grid(True, which='minor')
    ax.set_xlim([0, 200])
    ax.set_xticks([0, 1, 10, 100, 200])
    ax.xaxis.set_minor_locator(MinorSymLogLocator(1e-1))

signal_df2 = signal_df[signal_df.max(axis=0).sort_values(ascending=False).index.values].iloc[:, :10]

K562_polyA_TF_TPC_medians = K562_polyA_TF_TPC.replace(0, np.nan).median(axis=1).fillna(0)

# I want a nucleosome binding energy track on this figure

# chip peaks could be triangles or some other shape -- especially if we had the summit
# are the summits always in the middle?

# show the motif on the screen somewhere?
# fully featured genomics feature track?

# plot the problem statement

# maybe I should be plotting the TF's p-values rather than scores? coloring by correlation or something?
# TFBS: 2 colors: below threshold and above threshold

plot_chip_peaks = True
plot_chip_signal = True
plot_atac_signal = True
plot_tfbs = True
plot_dgf = True

num_rows = 1+len(signal_df2.columns)

plt.rcParams['figure.figsize'] = [18, num_rows]
plt.rcParams['path.simplify_threshold'] = 1.0

TF_names = signal_df2.columns.str.split('_').str[0].unique()
fig, axs = plt.subplots(len(TF_names), 2, sharex='col', gridspec_kw={'width_ratios': [3, 1]})
left_axs = [row[0] for row in axs]
row_dict = dict(zip(TF_names, axs[:-1]))


if plot_atac_signal:
    SPI1_promoter_TF_ATAC_signal.plot.line(ax=left_axs[-1], ylim=(0, SPI1_promoter_TF_ATAC_signal.max()), xlim=(start, stop), c='black', label='ATAC')
    left_axs[-1].set_ylabel('ATAC', rotation=0)
    plot_tss(left_axs[-1], tss, strand, axvline=True)
    style_ax(left_axs[-1])

for i, (TF_name, row) in enumerate(row_dict.items()):

    row[0].set_xlim([start, stop])
    row[0].set_ylim([0, 30])
    style_ax(row[0])
    color = 'darkblue' if i%2 == 0 else 'royalblue'

    for uid in signal_df2.loc[:, signal_df2.columns.str.split('_').str[0] == TF_name].columns:
        line = row[0].plot(signal_df2.index.values, signal_df2[uid].values, label=uid, clip_on=False, color=color, lw=1)
        # ax.legend(handles=line, loc='upper left', borderaxespad=0, frameon=False)

    g1 = sns.stripplot(data=K562_polyA_TF_TPC.loc[TF_name].to_frame(), x=TF_name, orient='h', alpha=0.5, c='lightblue', ax=row[1])
    g2 = sns.stripplot(data=K562_polyA_TF_TPC.loc[TF_name, best_polyA_files].to_frame(), x=TF_name, orient='h', color='red', ax=row[1])
    g3 = sns.stripplot(data=K562_polyA_TF_TPC_medians.to_frame().loc[[TF_name]].T, x=TF_name, orient='h', color='black', marker='D', ax=row[1])
    style_ax(row[1])
    row[1].set_xlim(0, K562_polyA_TF_TPC.max().max())
    row[1].set_ylabel(TF_name, rotation=0)
    # row[1].set_xscale('symlog', linthresh=1)
    # row[1].plot(K562_polyA_TF_TPC_medians.at[TF_name], TF_name, 'ko')
    if i == 0:
        row[1].spines['top'].set_visible(True)
        style_stripplot(row[1])

if plot_chip_peaks:
    for index, peak in peaks_df.iterrows():
        if peak['name'] in row_dict.keys():
            ax = row_dict[peak['name']][0]
            ax.add_patch(Rectangle((peak.chromStart, 0), peak.chromEnd-peak.chromStart, 30, fc=(1,0,0,np.clip(0.0005*peak.signalValue, a_min=0, a_max=1)), ec=(1,0,0,0.1), zorder=-1, clip_on=False))

if plot_tfbs:
    tfbs_cm = matplotlib.cm.ScalarMappable(norm=matplotlib.colors.Normalize(vmin=0, vmax=20), cmap=matplotlib.cm.OrRd)
    for index, tfbs in SPI1_cisbp_tfbs.iterrows():
        if tfbs['TFs'] in row_dict.keys():
            ax = row_dict[tfbs['TFs']][0]
            ax.add_patch(Rectangle((tfbs.start, 0), tfbs.end-tfbs.start, (tfbs.log10p-2)*4, fc=tfbs_cm.to_rgba(tfbs.log10p), ec=(0,0,0,0), zorder=10, clip_on=False))

            # fc=(0,1,0,np.clip(0.01*tfbs.log10p, a_min=0, a_max=1)),

if plot_dgf:
    last_ax = left_axs[-1]
    for index, dgf in k562_dgf.iterrows():
        alpha = 0.005*(dgf['K562-DS15363_posterior'] + dgf['K562-DS16924_posterior'])/2
        _,top = fig.transFigure.inverted().transform(left_axs[0].transAxes.transform([0,1]))
        _,bottom = fig.transFigure.inverted().transform(left_axs[-1].transAxes.transform([0,0]))
        trans = matplotlib.transforms.blended_transform_factory(left_axs[0].transData, fig.transFigure)
        fig.add_artist(Rectangle((dgf.core_start,bottom), dgf.core_end-dgf.core_start, top-bottom, transform=trans, fc=(0,0,1,alpha), ec=None, zorder=-10))

genomic_xticks(left_axs[-1], chrom=chrom)
axs[-1][1].axis('off')
axs[0][1].xaxis.set_tick_params(labeltop=True)

plt.savefig('/Users/alex/Desktop/SPI1_promoter_K562_TF_ChIP.png')
None
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-07-20T15:14:24.932483 image/svg+xml Matplotlib v3.5.2, https://matplotlib.org/
Scatterplots 
max_chip_peak_height = peaks_df.groupby('name')['signalValue'].max().rename('max_chip_peak_height')
max_chip_peak_height

name
ATF1        278.59146
ATF2        671.55342
ATF3       2017.85285
ATF4         61.95164
ATF7       1206.30707
              ...    
ZKSCAN1      28.54167
ZNF175       50.23833
ZNF281      112.63834
ZNF282       31.21194
ZNF766       56.29987
Name: max_chip_peak_height, Length: 87, dtype: float64
def set_colors(df, x_col, y_col):

    df['c'] = np.nan

    df.loc[(df[y_col] > 0) & (df[x_col] > 0), 'c'] = 'g'
    df.loc[(df[y_col] > 0) & (df[x_col] <= 0), 'c'] = 'm'
    df.loc[(df[y_col] <= 0) & (df[x_col] > 0), 'c'] = 'r'
    df.loc[(df[y_col] <= 0) & (df[x_col] <= 0), 'c'] = 'c'
    return df

K562_TF_RNA = pd.read_csv('../../back_of_my_envelope/data/equilibrium_TFs/K562_TF_RNA.csv', index_col=0)
K562_TF_RNA = K562_TF_RNA.replace(0, np.nan).median(axis=1).replace(np.nan, 0).rename('TF_RNA')
K562_TF_RNA

TFAP2A     0.082112
TFAP2B     0.019229
TFAP2C     0.024566
TFAP2D     0.000000
TFAP2E     0.029581
            ...    
MTERF1     8.131813
MTERF2    12.372807
TP53       2.256278
TP63       0.674158
TP73       0.592430
Name: TF_RNA, Length: 1635, dtype: float64
def process_peak_tfbs_df(tfbs_df):

    tfbs_df = tfbs_df[tfbs_df.TFs.isin(TFs_to_evaluate)]
    tfbs_df.p.clip(lower=1e-10, upper=None, inplace=True)
    tfbs_df['log10p'] = -np.log10(tfbs_df.p)
    tfbs_df = tfbs_df.merge(max_chip_peak_height, how='left', left_on='TFs', right_index=True)
    tfbs_df = tfbs_df.merge(K562_TF_RNA, how='left', left_on='TFs', right_index=True)
    tfbs_df['score_x_RNA'] = ln(exp(tfbs_df['score']) * tfbs_df['TF_RNA'])
    tfbs_df['log10p_x_RNA'] = tfbs_df['log10p'] * tfbs_df['TF_RNA']
    return tfbs_df.reset_index(drop=True)

    # distance to peak center
    # dgf

tfbs_df = process_peak_tfbs_df(SPI1_cisbp_tfbs)

def lims(series, buffer=0.01):

    spread = series.max() - series.min()
    limits = (series.min() - spread*buffer, series.max() + spread*buffer)
    return limits

def log_lims(series, log_buffer=1):

    lower = np.log10(series[series > 0].min()) - log_buffer
    upper = np.log10(series[series > 0].max()) + log_buffer
    return (lower, upper)

def spearman_corr(df, tfbs_col, chip_col): return df.loc[:, [tfbs_col, chip_col]].corr(method='spearman').loc[tfbs_col, chip_col]

spearman_corr(tfbs_df, 'score', 'max_signal'), spearman_corr(tfbs_df, 'score_x_RNA', 'max_signal')

(0.07881793222722186, 0.06831818983387146)
def plot_peak_scatter(df, mode='hist', x_col='score', y_col='max_signal', log=False, legend=False, ylim=(-0.1, 20)):

    palette={'g':'g','m':'m','r':'r','c':'c'}

    df = set_colors(df, x_col, y_col)
    df['chip_signalBinary'] = (df[y_col] > 0).astype(int)
    df['pwm_scoreBinary'] = (df[x_col] > 0).astype(int)

    g = sns.JointGrid(data=df, x=x_col, y=y_col, xlim=lims(df[x_col]), ylim=ylim, hue='c', palette=palette)
    g.plot_joint(sns.scatterplot, alpha=0.2, s=5, legend=legend)

    if mode == 'hist':
        sns.histplot(data=df, x=x_col, bins=100, ax=g.ax_marg_x, hue='chip_signalBinary', palette={0:'r', 1:'g'}, legend=False, log_scale=(False, True),element="step")
        if log:
            sns.histplot(data=df, y=y_col, bins=[0, 1]+np.logspace(1, 5).tolist(), ax=g.ax_marg_y, hue='pwm_scoreBinary', palette={0:'m', 1:'g'}, legend=False, log_scale=(True, False),element="step")
        else:
            sns.histplot(data=df, y=y_col, bins=200, ax=g.ax_marg_y, hue='pwm_scoreBinary', palette={0:'m', 1:'g'}, legend=False, log_scale=(True, False),element="step")

        g.ax_marg_y.tick_params(labeltop=True)
        g.ax_marg_y.grid(True, axis='x', ls=':')

        g.ax_marg_x.tick_params(labelleft=True)
        g.ax_marg_x.grid(True, axis='y', ls=':')

    elif mode=='kde':
        sns.kdeplot(data=df, x=x_col, ax=g.ax_marg_x, hue='chip_signalBinary', palette={0:'r', 1:'g'}, fill=True, alpha=0.1, legend=False)
        sns.kdeplot(data=df, y=y_col, ax=g.ax_marg_y, hue='pwm_scoreBinary', palette={0:'m', 1:'g'}, fill=True, alpha=0.1, legend=False, log_scale=(False, True))

    g.fig.set_figwidth(10)
    g.fig.set_figheight(5)

    corr = spearman_corr(df, x_col, y_col)
    g.fig.suptitle(f'Spearman_correlation: {round(corr, 4)}')

    if legend:
        handles, labels = g.ax_joint.get_legend_handles_labels()
        replacement = {'g':'Motif match and ChIP peak',
                       'm':'ChIP peak without motif match',
                       'r':'Motif match without ChIP peak',
                       'c':'Neither motif match nor ChIP Peak'}
        labels = [replacement[x] for x in labels]
        legend = g.ax_joint.legend(handles, labels, loc='upper left')

    if log:
        g.ax_joint.set_yscale('symlog', linthresh=min(0.1, df[df.max_signal > 0].max_signal.min()))

    return g, corr

g = plot_peak_scatter(tfbs_df, x_col='score', y_col='max_signal')

g = plot_peak_scatter(tfbs_df, x_col='score', y_col='max_chip_peak_height', log=True, ylim=(10, 1e3))

g = plot_peak_scatter(tfbs_df, x_col='score_x_RNA', y_col='max_signal')

g = plot_peak_scatter(tfbs_df, x_col='score_x_RNA', y_col='max_chip_peak_height', log=True, ylim=(10, 1e3))
Metrics 
 

tfbs_df
chrom start end name score strand TFs k562_chip_signal_files max_signal p log10p max_chip_peak_height TF_RNA score_x_RNA log10p_x_RNA c chip_signalBinary pwm_scoreBinary
0 chr11 47378177 47378186 M10306_2.00 5.672115 - IKZF1 ENCFF957MXZ 2.06838 0.000502 3.299445 NaN 36.913654 9.280697 121.794567 g 1 1
1 chr11 47378177 47378186 M10306_2.00 5.672115 - IKZF1 ENCFF053QLR 1.06389 0.000502 3.299445 NaN 36.913654 9.280697 121.794567 g 1 1
2 chr11 47378177 47378191 M04801_2.00 7.176316 + ETV5 ENCFF042WYK 1.48301 0.000069 4.161535 39.50612 9.482606 9.425776 39.462201 g 1 1
3 chr11 47378177 47378191 M09030_2.00 4.500186 + E2F1 ENCFF387AXO 0.68479 0.000608 3.216315 125.52399 8.737605 6.667822 28.102885 g 1 1
4 chr11 47378177 47378191 M09030_2.00 4.500186 + E2F1 ENCFF513XPM 0.91216 0.000608 3.216315 125.52399 8.737605 6.667822 28.102885 g 1 1
... ... ... ... ... ... ... ... ... ... ... ... ... ... ... ... ... ... ...
12235 chr11 47378961 47378972 M04401_2.00 3.655551 - KLF6 ENCFF955XUI 2.61929 0.000232 3.635227 NaN 5.719905 5.399503 20.793153 g 1 1
12236 chr11 47378961 47378973 M08281_2.00 0.209253 - ZNF586 ENCFF977XAH 1.42771 0.000318 3.497270 NaN 5.334281 1.883407 18.655423 g 1 1
12237 chr11 47378962 47378975 M09377_2.00 5.301170 - SMAD4 ENCFF836SJA 3.05519 0.000461 3.336172 119.26441 19.787000 8.286195 66.012841 g 1 1
12238 chr11 47378965 47378974 M03476_2.00 6.107840 + NFIX ENCFF431LIE 2.00159 0.000549 3.260517 NaN 3.746789 7.428739 12.216468 g 1 1
12239 chr11 47378965 47378974 M03477_2.00 5.603724 + NFIX ENCFF431LIE 2.00159 0.000844 3.073869 NaN 3.746789 6.924623 11.517139 g 1 1

12240 rows × 18 columns

def plot_distribs(no, yes, xlabel):

    fig, (ax0, ax1) = plt.subplots(1, 2)

    ax0 = no.plot.kde(ax=ax0, xlim=(yes.min(), yes.max()), legend=True)
    yes.plot.kde(ax=ax0, legend=True)

    ax0.set_xlabel(xlabel)

    no_x = no.sort_values()
    no_cdf = no_x.rank(method='average', pct=True)

    yes_x = yes.sort_values()
    yes_cdf = yes_x.rank(method='average', pct=True)

    ax1.plot([0]+no_x.tolist(), [0]+no_cdf.tolist(), label=no.name)
    ax1.plot([0]+yes_x.tolist(), [0]+yes_cdf.tolist(), label=yes.name)
    ax1.legend()
    ax1.set_ylim(0, 1)
    ax1.set_ylabel('CDF')
    ax1.set_xlabel(xlabel)

    return ax0, ax1

no = df[df.max_tfbs_score == 0].max_chip.rename('No TFBS')
yes = df[df.max_tfbs_score > 0].max_chip.rename('TFBS')
ax0, ax1 = plot_distribs(no, yes, 'ChIP signal')
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T10:29:47.874059 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/ 
no = df[df.max_chip == 0].max_tfbs_score.rename('No ChIP')
yes = df[df.max_chip > 0].max_tfbs_score.rename('ChIP')

ax0, ax1 = plot_distribs(no, yes, 'TFBS signal')
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T10:29:48.132447 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/ 
def PPV_TPR_FPR(df, thresh_index, tfbs='max_tfbs_score', chip='max_chip'):

    df.sort_values(tfbs, inplace=True)
    negative_prediction = df.iloc[:thresh_index]
    positive_prediction = df.iloc[thresh_index:]

    true_negative = sum(negative_prediction[chip] == 0)
    false_negative = sum(negative_prediction[chip] > 0)

    false_positive = sum(positive_prediction[chip] == 0)
    true_positive = sum(positive_prediction[chip] > 0)

    positive_predictive_value = (true_positive / (true_positive + false_positive)) if (true_positive + false_positive) > 0 else 1 # also called precision
    true_positive_rate = (true_positive / (true_positive + false_negative)) if (true_positive + false_negative) > 0 else 1        # also called recall
    false_positive_rate = (false_positive / (false_positive + true_negative)) if (false_positive + true_negative) > 0 else 1

    return positive_predictive_value, true_positive_rate, false_positive_rate

def rolling_PPV_TPR_FPR(df, tfbs='max_tfbs_score', chip='max_chip'):

    df.sort_values(tfbs, inplace=True)
    indices_of_thresholds = np.where(~df[tfbs].duplicated())[0]

    df = pd.DataFrame([PPV_TPR_FPR(df, thresh_index, tfbs=tfbs, chip=chip) for thresh_index in indices_of_thresholds], columns=['positive_predictive_value', 'true_positive_rate', 'false_positive_rate'])[::-1]

    df['f1'] = 2 * (df.positive_predictive_value * df.true_positive_rate) / (df.positive_predictive_value + df.true_positive_rate)

    return df

def AUROC(stats):

    auroc = np.trapz(stats.true_positive_rate, stats.false_positive_rate)
    return auroc

def plot_AUROC(stats):

    auroc = AUROC(stats)

    ax = stats.plot.line(y='true_positive_rate', x='false_positive_rate', legend=False, title=f'AUROC: {round(auroc, 3)}', xlim=(0, 1), ylim=(0, 1))
    ax.plot((0,1),(0,1), c='r', linestyle='dotted')

    ax.set_aspect('equal', adjustable='box')
    ax.set_ylabel('true_positive_rate')

    return ax, auroc

ax, auroc = plot_AUROC(rolling_PPV_TPR_FPR(df))
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T10:29:48.329299 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/

Generate reports for each peak 

plt.rcParams['figure.figsize'] = [12, 5]

peak_index = 9
df = K562_ATAC_2_tfbs_and_chip[peak_index]

def atac_peak_distrib_plot(peak_index, peaks_df=K562_ATAC_peaks_2_filtered):

    this_peak = peaks_df.loc[peak_index]

    g = sns.JointGrid(data=peaks_df, x='signalValue', y='score', xlim=(0,45), ylim=(0, 1050))
    g.plot_joint(sns.histplot)
    g.plot_marginals(sns.kdeplot)

    g.fig.suptitle(f'Peak signal percentile rank: {round(this_peak.signalValue_percentile*100, 2)}%')

    g.fig.set_figwidth(10)
    g.fig.set_figheight(5)

    g.ax_joint.plot(this_peak.signalValue, this_peak.score, markersize=12, c='red', marker="+", label=f'{peak_index}: '+this_peak['name'])
    g.ax_joint.plot(this_peak.signalValue, this_peak.score, markersize=10, c='red', marker=".")

    g.ax_marg_x.axvline(this_peak.signalValue, c='red')
    g.ax_marg_y.axhline(this_peak.score, c='red')

    g.ax_joint.legend(loc='lower right')

    return g

atac_peak_distrib_plot(peak_index)

<seaborn.axisgrid.JointGrid at 0x1b4ffe350>
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T10:30:04.337129 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/ 
def hypergeom_pval(df, universe=ChIPable_CISBPable_TFs, tfbs='pwm_score', chip='chip_signalValue'):

    TFs_with_chip_signal = set(df[df[chip] > 0].index)
    TFs_with_tfbs = set(df[df[tfbs] > 0].index)

    # the total number of marbles in the jar
    M = len(ChIPable_CISBPable_TFs)

    # the number of red marbles in the jar
    n = len(TFs_with_chip_signal)

    # the number of draws from the jar
    N = len(TFs_with_tfbs)

    # the number of red marbles drawn
    x = len(TFs_with_chip_signal & TFs_with_tfbs)

    pval = hypergeom.sf(x-1, M, n, N)

    return pval


def pwm_chip_overlap_venn(df):

    fig, ax = plt.subplots()

    TFs_with_chip_signal = set(df[df.chip_signalValue > 0].index)
    TFs_with_tfbs = set(df[df.pwm_score > 0].index)

    pval = hypergeom_pval(df)

    venn3((set(ChIPable_CISBPable_TFs), TFs_with_tfbs, TFs_with_chip_signal),
          (f'All TFs we have PWMs and ChIP data for ({len(ChIPable_JASPARable_TFs)})',
           f'Has non-zero PWM score at locus ({len(TFs_with_tfbs)})',
           f'Has non-zero ChIP signal at locus ({len(TFs_with_chip_signal)})'))

    fig.suptitle(f'Hypergeometric -log10(pval): {round(-np.log10(pval),2)}')

    return fig, pval

ax, pval = pwm_chip_overlap_venn(df)
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T13:43:34.812882 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/ 
TFs_with_SPI1_promoter_chip_signal = set(tfbs_df[tfbs_df.max_chip_peak_height.notnull()].TFs)
TFs_with_SPI1_promoter_tfbs = set(tfbs_df.TFs)

plt.rcParams['figure.figsize'] = [12, 5]

venn_obj = venn3((TFs_with_SPI1_promoter_tfbs, set(TFs_to_evaluate), TFs_with_SPI1_promoter_chip_signal),
                  (f'Has non-zero PWM score at locus ({len(TFs_with_SPI1_promoter_tfbs)})',
                   f'All TFs we have PWMs and ChIP data for ({len(TFs_to_evaluate)})',
                   f'Has a ChIP peak at locus ({len(TFs_with_SPI1_promoter_chip_signal)})'))

for text in venn_obj.set_labels: text.set_fontsize(8)
for text in [x for x in venn_obj.subset_labels if x]: text.set_fontsize(6)

stats = rolling_PPV_TPR_FPR(df, tfbs='pwm_score', chip='chip_signalValue').iloc[1:]

def plot_PR(stats):

    stats = stats.rename(columns={'positive_predictive_value':'precision', 'true_positive_rate': 'recall'})
    ax = stats.plot.line(y='precision', x='recall', legend=False, xlim=(0, 1), ylim=(0, 1))
    ax.set_aspect('equal', adjustable='box')
    ax.set_ylabel('precision')
    ax.set_xlabel('recall')

    f_scores = np.linspace(0.2, 0.8, num=4)
    lines, labels = [], []
    for f_score in f_scores:
        x = np.linspace(0.01, 1)
        y = f_score * x / (2 * x - f_score)
        (l,) = plt.plot(x[y >= 0], y[y >= 0], color="gray", alpha=0.2)
        plt.annotate("f1={0:0.1f}".format(f_score), xy=(0.9, y[45] + 0.02))

    top_f1 = stats.f1.max()
    ax.set_title(f'Best F1 score: {round(top_f1, 2)}')

    return ax, top_f1

ax, top_f1 = plot_PR(stats)
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T13:43:36.101047 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/ 
def peak_summary(peak_index):

    peak_df = clean_peak_df(K562_ATAC_2_tfbs_and_chip[peak_index])

    g1 = atac_peak_distrib_plot(peak_index)

    g2 = plot_peak_scatter(peak_df)

    ax, hypergeom_pval = pwm_chip_overlap_venn(peak_df)

    stats = rolling_PPV_TPR_FPR(peak_df, tfbs='pwm_score', chip='chip_signalValue').iloc[1:]
    ax, top_f1 = plot_PR(stats)
    ax, auroc = plot_AUROC(stats)

    return peak_df

peak_df = peak_summary(9)
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T13:43:37.426045 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T13:43:38.020791 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T13:43:38.148149 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T13:43:38.217033 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-04-07T13:43:38.290944 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/ 
def detailed_peak_summary():
    pass

Evaluating across all ATAC peaks 

# by peak
# by motif
# by tf

def evaluate_predictor(df, x_col_name, y_col_name):

    num_nonzero_predictions
    num_nonzero_ground_truth

    corr = spearman_corr(df[x_col_name], df[y_col_name])

    stats = rolling_PPV_TPR_FPR(peak_df, tfbs=x_col_name, chip=y_col_name).iloc[1:]
    top_f1 = stats.f1.max()
    auroc = AUROC(stats)

    return (atac_signalValue_percentile,
            num_TFs_overall,
            num_chip_peaks,
            num_tfbs_hits,
            num_phantom_tfs,
            corr_without_rna,
            corr_with_rna,
            pval,
            top_f1,
            auroc)

 

def peak_stats(peak_index):

    atac_signalValue_percentile = K562_ATAC_peaks_2_filtered.loc[peak_index].at['signalValue_percentile']

    peak_df = clean_peak_df(K562_ATAC_2_tfbs_and_chip[peak_index])

    num_TFs_overall = len(peak_df)
    num_chip_peaks = peak_df.chip_signalBinary.sum()
    num_tfbs_hits = peak_df.pwm_scoreBinary.sum()
    num_phantom_tfs = len(peak_df[(peak_df.TF_conc == 0) & (peak_df.chip_signalValue > 0)])

    corr_without_rna = spearman_corr(peak_df, 'pwm_score', 'chip_signalValue')
    corr_with_rna = spearman_corr(peak_df, 'pwm_score_x_TF_conc', 'chip_signalValue')

    pval = hypergeom_pval(peak_df)

    stats = rolling_PPV_TPR_FPR(peak_df, tfbs='pwm_score', chip='chip_signalValue').iloc[1:]
    top_f1 = stats.f1.max()
    auroc = AUROC(stats)

    return (atac_signalValue_percentile,
            num_TFs_overall,
            num_chip_peaks,
            num_tfbs_hits,
            num_phantom_tfs,
            corr_without_rna,
            corr_with_rna,
            pval,
            top_f1,
            auroc)

#         'num_TFs_overall',
#         'num_chip_peaks',
#         'num_tfbs_hits',
#         'num_phantom_tfs',
#         'corr_without_rna',
#         'corr_with_rna',
#         'pval',
#         'top_f1',
#         'auroc')

# metrics_df = {i: peak_stats(i) for i in K562_ATAC_2_tfbs_and_chip.keys()}
# metrics_df = pd.DataFrame(metrics_df, index=cols).T
# metrics_df.to_csv('../data/equilibrium_TFs/K562_ATAC_PWM_vs_ChIP_baseline_metrics.csv')
metrics_df = pd.read_csv('../data/equilibrium_TFs/K562_ATAC_PWM_vs_ChIP_baseline_metrics.csv', index_col=0)

metrics_df = pd.concat((K562_ATAC_peaks_2_filtered.signalValue, metrics_df), axis=1).rename(columns={'signalValue':'atac_signalValue'})

set_matplotlib_formats('png')
metrics_df.plot.scatter(x='atac_signalValue', y='num_chip_peaks', alpha=0.3, s=0.1)

<AxesSubplot:xlabel='atac_signalValue', ylabel='num_chip_peaks'>

Per TF, Motif 

# engine = create_engine('sqlite:////Users/alex/Documents/back_of_my_envelope/data/sqlite.db', echo=False)
# with engine.begin() as connection:
#     for key, df in cisbp2_vs_chip:
#         df['key'] = key
#         df.to_sql('cisbp2_vs_chip', connection, if_exists="append", index=False)

intervals_df = pd.read_csv('/Users/alex/Documents/AChroMap/scripts/K562_ATAC_peaks_1.csv', header=None)

def _key(chrom, start, stop): return f'/{chrom}/{start}-{stop}'

K562_TF_RNA = pd.read_csv('../../back_of_my_envelope/data/equilibrium_TFs/K562_TF_RNA.csv', index_col=0)
K562_TF_RNA = K562_TF_RNA.replace(0, np.nan).median(axis=1).replace(np.nan, 0).rename('TF_RNA')
K562_TF_RNA

TFAP2A     0.082112
TFAP2B     0.019229
TFAP2C     0.024566
TFAP2D     0.000000
TFAP2E     0.029581
            ...    
MTERF1     8.131813
MTERF2    12.372807
TP53       2.256278
TP63       0.674158
TP73       0.592430
Name: TF_RNA, Length: 1635, dtype: float64
def get_K562_TF_ChIP_peaks(chrom, start, end):

    k562_merged_ChIP_path = '/Users/alex/Documents/AChroMap/data/raw/ENCODE/k562_chip/K562_ChIP_merged.bed.gz'
    tabix_coords = chrom+':'+str(start)+'-'+str(end)
    tabix_command = f'tabix {k562_merged_ChIP_path} {tabix_coords}'
    peaks_contained_in_region = read_shell(shlex.split(tabix_command), sep='\t', header=None, names=['chrom','chromStart','chromEnd','name','signalValue'])

    return peaks_contained_in_region

def process_peak_tfbs_df(tfbs_df):

    tfbs_df = tfbs_df[tfbs_df.TFs.isin(TFs_to_evaluate)]
    tfbs_df.p.clip(lower=1e-10, upper=None, inplace=True)
    tfbs_df['log10p'] = -np.log10(tfbs_df.p)
    # tfbs_df = tfbs_df.merge(max_chip_peak_height, how='left', left_on='TFs', right_index=True)
    tfbs_df = tfbs_df.merge(K562_TF_RNA, how='left', left_on='TFs', right_index=True)
    tfbs_df['score_x_RNA'] = ln(exp(tfbs_df['score']) * tfbs_df['TF_RNA'])
    tfbs_df['log10p_x_RNA'] = tfbs_df['log10p'] * tfbs_df['TF_RNA']
    return tfbs_df.reset_index(drop=True)

    # distance to peak center
    # dgf

def spearman_corr(df, x_col, y_col): return df.loc[:, [x_col, y_col]].corr(method='spearman')[x_col].get(y_col, np.nan)

len(TFs_to_evaluate)

225
with open('/Users/alex/Desktop/corrs.pickle', 'rb') as pickle_f:
    corrs = pickle.load(pickle_f)

overlaps_promoters = pd.read_csv('/Users/alex/Desktop/test1.txt', header=None)

overlaps_promoters.value_counts()

0    67053
dtype: int64

Probably no longer relevant:

Let's now proceed to a slightly more complex regulatory region, with 20 binding sites for 10 TFs

<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-02-04T11:37:39.954187 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/

Notice first that when binding sites overlap slightly, we've introduced a free energy penalty of competition, denoted by a pink line between the binding sites, and when they stronly overlap, we've disallowed those configurations, denoted by a red line.

We notice a problem with evaluating our $p_\mathrm{config}$ expression with realistic regulatory DNA: the number of configurations grows exponentially in the number of transcription factor binding sites ($2^{|\mathrm{TFBS}|}$). Only 20 Transcription Factor Binding sites entails ~1 million configurations, and so ~1 million terms in the denominator $Z$. Computing probabilities of configurations exactly then becomes intractable for realistic scenarios: we need to proceed by sampling.

Thankfully there's a dynamic programming approach to computing Z.

config = np.round(np.random.rand(len(TFBSs))).astype(int)

Which we can plot, and compute the associated log-statistical-weight:

axs = draw_config(config)
plt.tight_layout()
print('log(p_config) =', log_P_config(config))

log(p_config) = -1.4748275325663975
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-02-03T18:15:21.883707 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/

References

Multiple TFs: Nucleosome-mediated Cooperativity

nucleosomes as a soft AND gate

Direct cooperativity (driven by protein-protein interactions) between transcription factors exerts significant control over transcription when it occurs, but it appears to be the exception, not the rule. More common is indirect cooperativity mediated by nucleosomes. Nucleosomes can be thought of as repressive transcription factors with large footprints and relatively non-specific sequence-dependent binding affinities. When multiple transcription factors bind independently in the footprint of a nucleosome, they do so cooperatively, despite not making physical contact with one another.

Including nucleosomes in our description of chromatin produces a new set of interactions, which require energy parameters. Those are:

  • Nucleosome:DNA interactions
  • Nucleosome:TF interactions
  • Nucleosome:Nucleosome interactions

Nucleosome:DNA

Nucleosomes have at least a 5000-fold range of affinities for differing DNA sequences.

What controls nucleosome positions? Nucleosomes do not bind specific 147-bp sequences, but they do have preferences for certain periodic patterns, and disfavor certain other patterns. Nucleosomes are canonically wrapped by 147bp of DNA, but due to nucleosome crowding and nucleosome beathing, they are often wrapped by fewer bases.

For our model, we will say that any stretch of DNA [of lengths 100-147bp] can be wrapped by a nucleosome, with an energy which is derived from the [sequence model here].

def nucleosome_energy_from_sequence(sequence):
    '''
    tells you the binding energy
    '''
    pass

Nucleosome:TF

Nucleosomes and Transcription Factors were considered to sterically block one another from binding the same stretch of DNA, however, we now know that many TFs can co-bind DNA and stabilize nucleosomal binding to a piece of DNA.

For simplicity, we'll assume we're only considering transcription factors which cannot co-bind DNA with nucleosomes, and impose a large ΔG penalty for nucleosome and TF co-binding.

Nucleosome:Nucleosome

Nucleosomes are spaced by stretches of free DNA called linker DNA, which have characterisitic lengths. Those specific lengths are believed to facilitate nucleosome arrays forming higher-order chromatin structures.

def energy_of_spacing_between_nucleosomes(nucleosome_positions):
    '''
    '''
    pass

nanomolar_kd_from_kcal_ΔG(23.8*kbT)

0.04610959744808222
nanomolar_kd_from_kcal_ΔG(14.4*kbT)

557.3903692694596
# Resgen: chip, dgf, and atac
# chip: bigwig, bed
# dgf: bed
# atac: bigwig, bed

send blog post for feedback:

  • Jeremy / Leonid
  • Advait / Bin Zhang
  • Anders
  • Kellis lab
  • Vlad Teif, other online nucleosome people
  • TF people?
  • Muir Morrison / Rob Philips
  • Bruce Tidor?

transfer matrix: probably won't use 

# the PWMs are concatenated head to tail on the y/i axis (and expanded ACGT). The next base is on the x/j axis: there are 4 values in the row.

# the start of each motif is that value * concentration

# I need hypothetical absolute max kd's ΔG's. Then everything is relative to that. Does the transfer matrix sum energies or something else?

# how does the particular sequence enter the matrix multiplication? You're choosing a path through the matrix.
# Does that mean the matrix at each base is different? Or there are 4 matrices again?
# yeah I think there have to be 4 matrices (or 6 if you include methylation). Wait since matrices are for each pair of positions, we need 16 acctually?

sum(tfdb.log_pwm.apply(len))

12757
transfer_matrix_a = np.zeros((len(tf_states), len(tf_states)))
# transfer_matrix_c = np.zeros((len(tf_states), len(tf_states)))
# transfer_matrix_g = np.zeros((len(tf_states), len(tf_states)))
# transfer_matrix_t = np.zeros((len(tf_states), len(tf_states)))

tfdb.log_pwm

TFAP2A    [[-0.5324, 0.34450000000000003, -1.5585, 0.530...
TFAP2B    [[-2.4892, 0.5962000000000001, 1.0115, -2.6127...
TFAP2C    [[-0.5975, -0.163, -1.1323, 0.7243], [-1.3232,...
TFAP2D    [[0.8455, -1.4861, 0.1063, -1.8089], [-5.6699,...
TFAP2E    [[-5.6699, 0.43660000000000004, 1.1706, -5.669...
                                ...                        
BATF3     [[-0.8147000000000001, 0.11900000000000001, 0....
CREB1     [[-0.049100000000000005, -0.20500000000000002,...
TP53      [[0.1729, -0.6791, 0.6519, -0.661], [0.7944, -...
TP63      [[-0.5391, -1.3228, 1.0218, -0.394300000000000...
TP73      [[0.08610000000000001, -0.6413, 0.738200000000...
Name: log_pwm, Length: 1080, dtype: object
base_index = {'a':0,'c':1,'g':2,'t':3}

tf_states = pd.Index([(tf, i) for tf, log_pwm in tfdb.log_pwm.items() for i in range(len(log_pwm))])

def transfer_matrix_index(TF, offset): return tf_states.get_loc((TF, offset))

for TF, pwm in tfdb.log_pwm.items():
    for offset, row in enumerate(pwm):

        i = transfer_matrix_index(TF, offset)

        transfer_matrix_a[i][i+1] = pwm[offset][base_index['a']]

----------------------------------------------------------
IndexError               Traceback (most recent call last)
<ipython-input-231-ffe1fe50396a> in <module>
      4         i = transfer_matrix_index(TF, offset)
      5 
----> 6         transfer_matrix_a[i][i+1] = pwm[offset][base_index['a']]
      7 

IndexError: index 12757 is out of bounds for axis 0 with size 12757

Verify against proteomics 

df = pd.read_csv('https://gygi.hms.harvard.edu/data/ccle/protein_quant_current_normalized.csv.gz')

df = df[['Protein_Id',
         'Gene_Symbol',
         'Description',
         'Group_ID',
         'Uniprot',
         'Uniprot_Acc',
         'K562_HAEMATOPOIETIC_AND_LYMPHOID_TISSUE_TenPx25']].rename(columns={'K562_HAEMATOPOIETIC_AND_LYMPHOID_TISSUE_TenPx25':'Gygi_K562'})

updates, missing, names_updated = namespace_mapping(df.Gene_Symbol)

passed 12755 symbols
31 names contained whitespace. Stripping...
558 duplicates. 12197 uniques.

querying 1-1000...done.
querying 1001-2000...done.
querying 2001-3000...done.
querying 3001-4000...done.
querying 4001-5000...done.
querying 5001-6000...done.
querying 6001-7000...done.
querying 7001-8000...done.
querying 8001-9000...done.
querying 9001-10000...done.
querying 10001-11000...done.
querying 11001-12000...done.
querying 12001-12197...done.
Finished.
728 input query terms found dup hits:
	[('ARX', 2), ('NCL', 2), ('CNP', 2), ('RPF1', 2), ('HYPK', 2), ('FH', 3), ('RPL17', 3), ('ATF2', 2),
13 input query terms found no hit:
	['FLJ22184', 'L1RE1', 'MT-ND5', 'MT-ND4', 'MT-CO1', 'MT-CYB', 'MT-ND1', 'GAGE3', 'MT-ATP8', 'hCG_198

unchanged: 11537;  updates: 647;  missing: 13

df.Gene_Symbol = names_updated

k562_TF_proteomics = df.groupby('Gene_Symbol')['Gygi_K562'].max().reindex(tfdb.index)

sum(k562_TF_proteomics.notnull()) / len(k562_TF_proteomics)

0.387037037037037
k562_TF_proteomics

TFAP2A   -2.802736
TFAP2B   -2.548439
TFAP2C   -3.410715
TFAP2D         NaN
TFAP2E         NaN
            ...   
BATF3          NaN
CREB1     0.484013
TP53     -3.214319
TP63     -0.861330
TP73           NaN
Name: Gygi_K562, Length: 1080, dtype: float64
tf_proteomics_validation = pd.concat((K562_TF_nanomolar_conc.rename('[TF] predicted from ENCODE RNA'), k562_TF_proteomics), axis=1)
tf_proteomics_validation
[TF] predicted from ENCODE RNA Gygi_K562
TFAP2A 0.519441 -2.802736
TFAP2B 0.121646 -2.548439
TFAP2C 0.155407 -3.410715
TFAP2D 0.000000 NaN
TFAP2E 0.187127 NaN
... ... ...
BATF3 3.407311 NaN
CREB1 80.409642 0.484013
TP53 14.273234 -3.214319
TP63 4.264729 -0.861330
TP73 3.747720 NaN

1080 rows × 2 columns

supp3 = pd.read_excel('~/Desktop/geiger_supp/mcp.M111.014050-3.xlsx', skiprows=[0])
supp3 = supp3[['Protein IDs', 'Protein Names', 'Gene Names', 'Uniprot', 'Peptides','Razor + unique Peptides', 'Unique Peptides', 'Sequence Coverage [%]','Mol. Weight [kDa]', 'PEP', 'iBAQ K562_1','iBAQ K562_2', 'iBAQ K562_3','LFQ Intensity K562_1', 'LFQ Intensity K562_2', 'LFQ Intensity K562_3',]]

supp3['Gene Names'] = supp3['Gene Names'].str.split(';')

sum(supp3['Gene Names'].isna()) / len(supp3)

0.060758414997869624
supp3_genes = [x for l in supp3['Gene Names'].values if str(l) != 'nan' for x in l]

updates, missing, names_updated = namespace_mapping(supp3_genes)

passed 30872 symbols
2285 duplicates. 28587 uniques.

querying 1-1000...done.
querying 1001-2000...done.
querying 2001-3000...done.
querying 3001-4000...done.
querying 4001-5000...done.
querying 5001-6000...done.
querying 6001-7000...done.
querying 7001-8000...done.
querying 8001-9000...done.
querying 9001-10000...done.
querying 10001-11000...done.
querying 11001-12000...done.
querying 12001-13000...done.
querying 13001-14000...done.
querying 14001-15000...done.
querying 15001-16000...done.
querying 16001-17000...done.
querying 17001-18000...done.
querying 18001-19000...done.
querying 19001-20000...done.
querying 20001-21000...done.
querying 21001-22000...done.
querying 22001-23000...done.
querying 23001-24000...done.
querying 24001-25000...done.
querying 25001-26000...done.
querying 26001-27000...done.
querying 27001-28000...done.
querying 28001-28587...done.
Finished.
1899 input query terms found dup hits:
	[('GJC1', 2), ('ATP6C', 2), ('A15', 2), ('MYPOP', 2), ('LAG1', 4), ('LASS1', 2), ('UOG1', 2), ('SDP1
8595 input query terms found no hit:
	['FAM1B', 'DKFZp686P0738', 'hCG_23354', 'KIAA0693', 'HSPC198', 'BM-004', 'NKD', 'PP7246', 'hCG_18772

unchanged: 9507;  updates: 10576;  missing: 8595

supp3['gene_name'] = [[] for _ in range(len(supp3))]

for i, names in supp3['Gene Names'].items():
    if str(names) != 'nan':
        for name in names:
            if name in updates:
                supp3.iloc[i].gene_name.append(updates[name])
            elif name not in missing:
                supp3.iloc[i].gene_name.append(name)

supp3['ambiguous'] = False

for i, names in supp3['gene_name'].items():
    if len(names) == 0:
        supp3.loc[i, 'gene_name'] = np.nan
    elif all(name == names[0] for name in names):
        supp3.loc[i, 'gene_name'] = names[0]
    else:
        supp3.loc[i, 'ambiguous'] = True

len(supp3[supp3.ambiguous]) / len(supp3)

0.11231359181934385
Mann_K562 = supp3[supp3.gene_name.isin(tfdb.index)].set_index('gene_name').drop('Gene Names', axis=1)
Mann_K562 = Mann_K562[['LFQ Intensity K562_1','LFQ Intensity K562_2','LFQ Intensity K562_3']].rename(columns={'LFQ Intensity K562_1':'Mann_K562_1','LFQ Intensity K562_2':'Mann_K562_2','LFQ Intensity K562_3':'Mann_K562_3'})

Mann_K562 = Mann_K562.reset_index().iloc[Mann_K562.notnull().sum(axis=1).reset_index().groupby('gene_name')[0].idxmax().values].set_index('gene_name').reindex(tfdb.index)
Mann_K562 = Mann_K562.median(axis=1)

Mann_K562

TFAP2A   NaN
TFAP2B   NaN
TFAP2C   NaN
TFAP2D   NaN
TFAP2E   NaN
          ..
BATF3    NaN
CREB1    NaN
TP53     NaN
TP63     NaN
TP73     NaN
Length: 1080, dtype: float64
tf_proteomics_validation = pd.concat((tf_proteomics_validation, Mann_K562.rename('Mann_K562')), axis=1)

tf_proteomics_validation.plot.scatter(x='[TF] predicted from ENCODE RNA', y='Gygi_K562', logx=True)

<AxesSubplot:xlabel='[TF] predicted from ENCODE RNA', ylabel='Gygi_K562'>
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-02-24T18:38:31.021492 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/ 
tf_proteomics_validation.plot.scatter(x='[TF] predicted from ENCODE RNA', y='Mann_K562', logx=True)

<AxesSubplot:xlabel='[TF] predicted from ENCODE RNA', ylabel='Mann_K562'>
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-02-24T18:39:25.544396 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/ 
tf_proteomics_validation.plot.scatter(x='Gygi_K562', y='Mann_K562')

<AxesSubplot:xlabel='Gygi_K562', ylabel='Mann_K562'>
<!DOCTYPE svg PUBLIC "-//W3C//DTD SVG 1.1//EN" "http://www.w3.org/Graphics/SVG/1.1/DTD/svg11.dtd"> 2022-02-24T18:39:23.630016 image/svg+xml Matplotlib v3.5.1, https://matplotlib.org/